Question

What is the distance to the horizon from this point?

A person standing at the top of Mountain Aconcagua would be approximately 4.3 mi high. The radius of earth is 3959 mi.
What is the distance to the horizon from this point?
Enter your answer as a decimal in the box. Round only your final answer to the nearest tenth.

Answer 1

The distance to the horizon is `"184.6 mi"`.

Explanation:

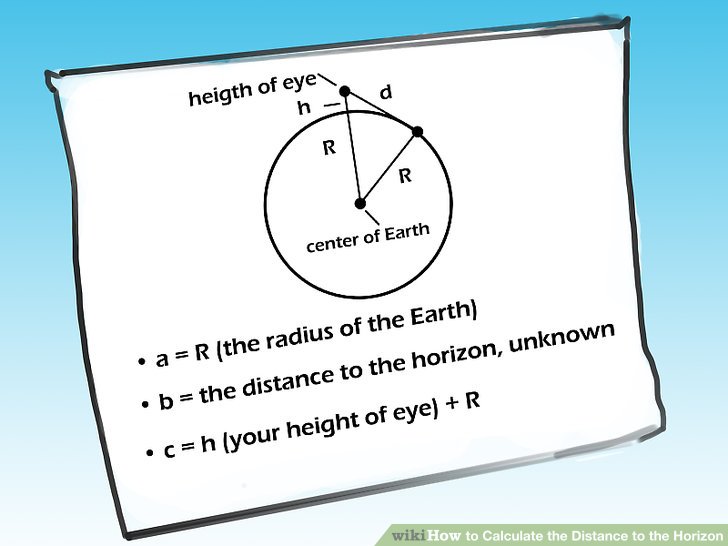
The diagram shows how the height of the person and mountain, the radius of the earth, and the distance to the horizon relate to the sides of a right triangle.

Side `a` is the radius of the earth.

`a` `=` `"3959 mi"`

Side `c`, the hypotenuse, is the sum of the radius of the earth and the height of the person and mountain.

`c` `=` `"3959 mi + 4.3 mi"`

`c` `=` `"3963.3 mi"`

Use the Pythagorean theorem to find side `"b"`, which is the distance to the horizon.

`c^2=a^2+b^2`

`b^2=c^2-a^2`

`b=sqrt(c^2-a^2)`

Plug in the known values.

`b=sqrt(("3963.3 mi")^2-(3959)^2)`

`b="184.6 mi"`

The distance to the horizon is `"184.6 mi"`.

Note: In the diagram below, the `d` in the diagram represents side `b` of a right triangle. Also, this diagram used the height of the person's eyes as the point of measurement. That was not a part of this question and you can ignore that.