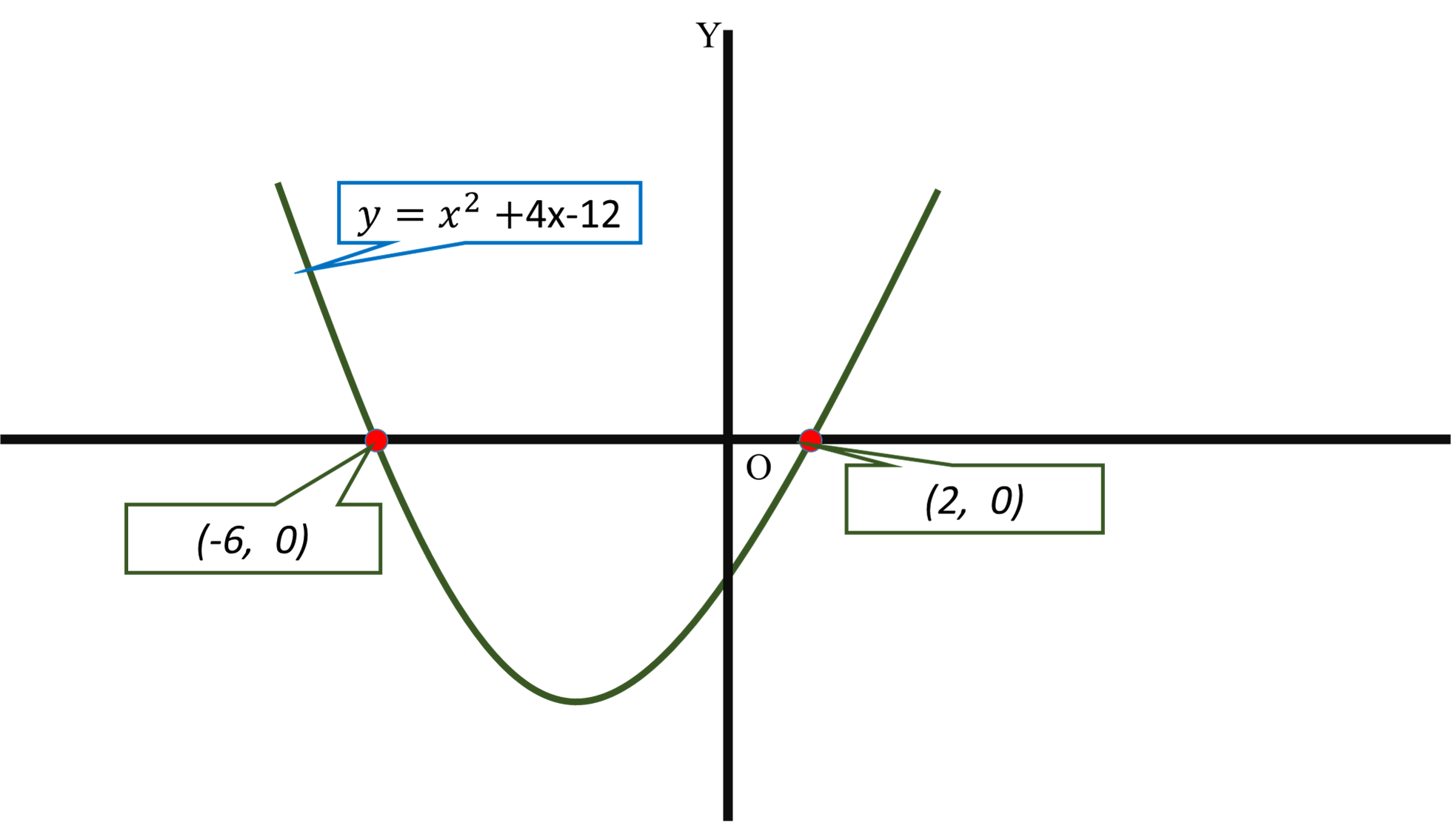
What is the equation of a parabola that opens upward and has #x#-intercepts at #(2,0)# and #(-6,0)#?

Question

4951 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-09-28T06:09:31

#y=x^2+4x-12#

It is a parabola.

Its x-intercepts are #(-6, 0) and (2, 0)#

Equation can be formed like this -

#x=-6#
#x+6=0#
#(x+6)# is one of the factors of quadratic equation.

#x=2#
#x-2=0#
#x-2=0# is another factor of quadratic equation.

The quadratic function is -

#y=(x+6)(x-2)#

#y=x^2+6x-2x-12#

#y=x^2+4x-12#

Look at the graph