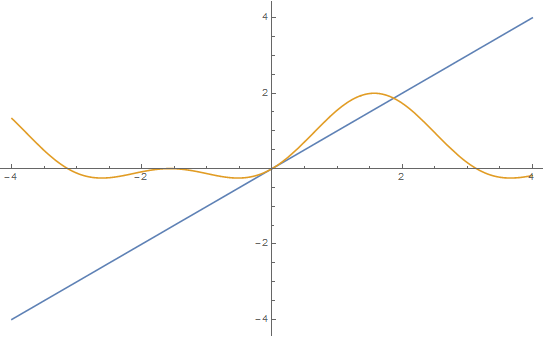Question

What is the equation of the tangent line of `f(x)=sin x + sin^2 x` at `x=0`?

Answer 1

`y=x`

Explanation:

This problem can be tackled in 3 steps:

1. Figure out the gradient of the line using the derivative of the function and the given `x` value.
2. Use the given `x` value and `f(x)` to find `C`.
3. Put values into the standard equation of a straight line.

The equation of a line `-> y = mx+C`

1. We'll begin by working out `m`, the gradient of the tangent. To do this we simply have to find `f'(x)`, the derivative.

`f(x) = sin(x) + sin^2(x)`
`f'(x) = cos(x) + 2cos(x)sin(x)`

Where the chain rule has been used on the `sin^2(x)` term.

Since we want to find the tangent at `x=0` then the gradient can be given by:
`f'(0)`
`=cos(0)+2cos(0)sin(0) = 1`

Thus `m=1`.
Step 1 is complete.

2. We must now find `C`.

Use `f(0)`
`=sin(0)+sin^2(0)=0`

Thus `{0,0}` Are the co -ordinates which the line intercepts `f(x)` so we know the line passes through `{0,0}`.

So, using `y = 0, x = 0 and m=1` we can substitute these into our equation of a straight line to obtain:

`y = mx + C`
`(0) = (1)(0) +C`
`C=0`

Thus we have our value for `C`. Step 2 is complete.

3. We can now write:

`y = mx+C`
`y = 1(x)+(0)` So the final equation is:
`y = x`

Indeed we see that if we plot both on the same graph we get a line intersection at a tangent exactly where we would expect: