Question

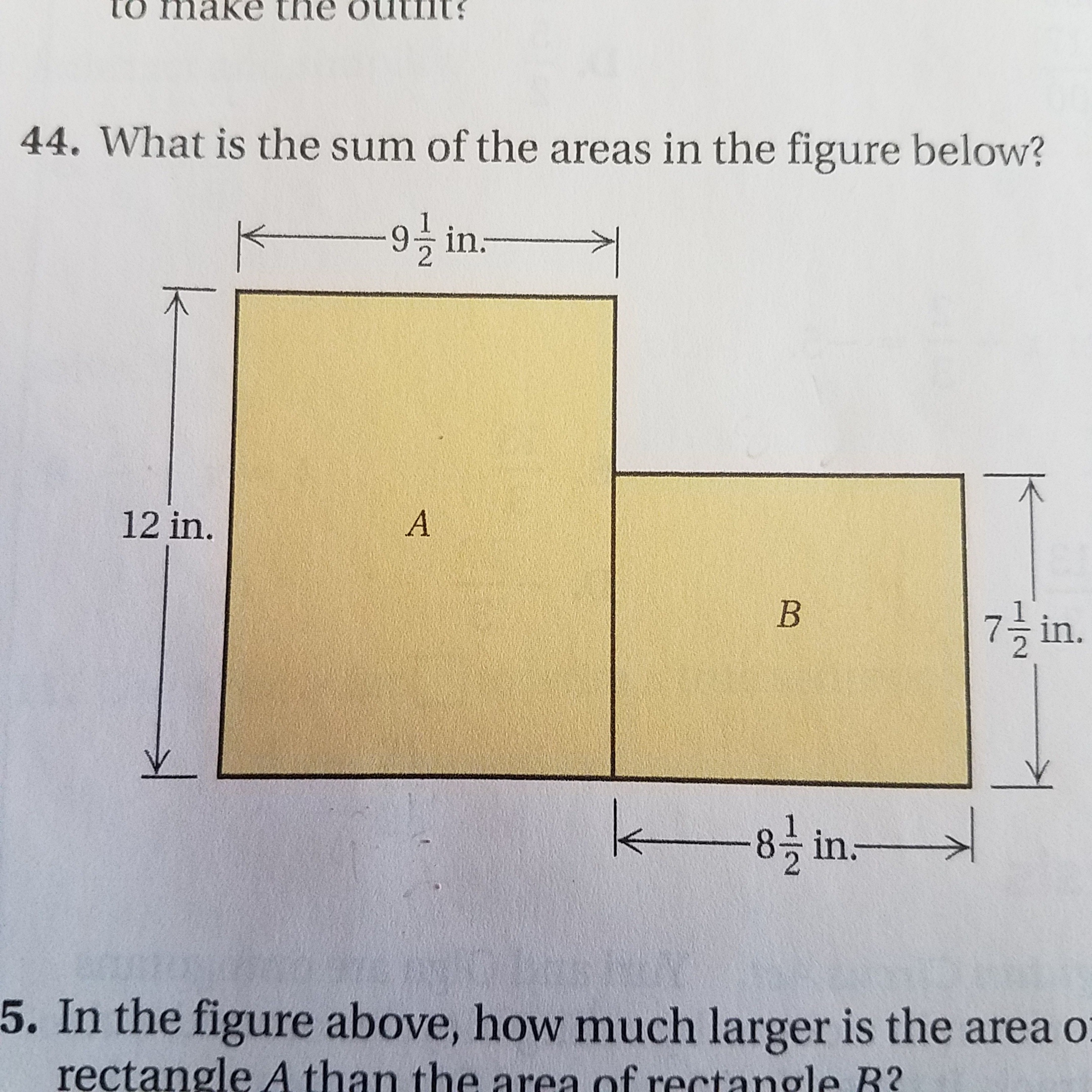
What is the sum of the area in the figure below?

Answer 1

See a solution process below:

Explanation:

Formula for the area of rectangle is:

`A = l xx w`

We can convert the dimensions in the problem which are mixed numbers to improper fractions for easier multiplication:

`9 1/2 = 9 + 1/2 = (2/2 xx 9) + 1/2 = 18/2 + 1/2 = 19/2`

`8 1/2 = 8 + 1/2 = (2/2 xx 8) + 1/2 = 16/2 + 1/2 = 17/2`

`7 1/2 = 7 + 1/2 = (2/2 xx 7) + 1/2 = 14/2 + 1/2 = 15/2`

The area of rectangle A is:

`A_a = 12"in" xx 19/2"in" = 228/2"in"^2 = 114"in"^2`

The area of rectangle B is:

`A_b = 17/2"in" xx 15/2"in" = 255/2"in"^2 =`

`(254 + 1)/2"in" = (254/2 + 1/2)"in"^2 = (127 + 1/2)"in"^2 =`

`127 1/2"in"^2`

To find the sum of the area in the problem we add the area of rectangle A and Rectangle B giving:

`A_a + A_b = 114"in"^2 + 127 1/2"in"^2 = 241 1/2"in"^2`