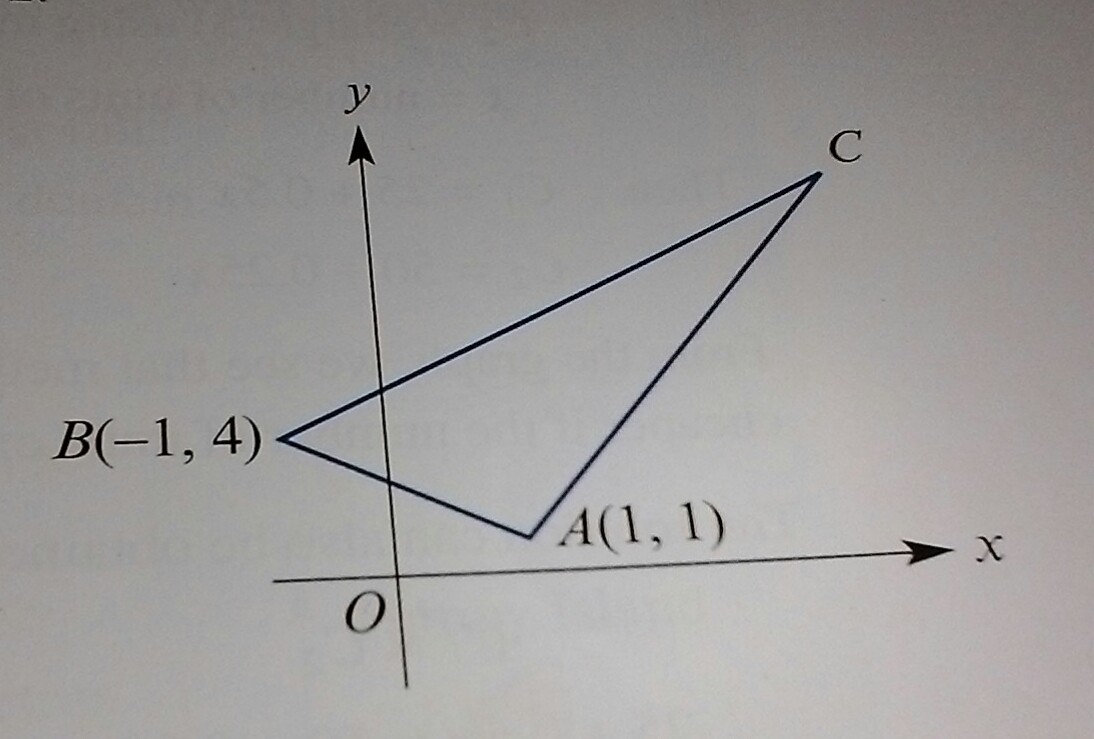
What is the value of m and the coordinates of C?

enter image source here
enter image source here

1 Answer
CW
Jan 29, 2018

see explanation.

Explanation:

enter image source here
let #m_(AB), m_(AC) and m_(BC)# be the slope of #AB, AC and BC#, respectively.
a) #m_(AB)=(4-1)/(-1-1)=-3/2#
given #m_(AB)=-3m, => -3m=-3/2, => m=1/2#
#=> m_(AC)=3m=3/2, and m_(BC)=m=1/2#

b) let #(x,y)# be the coordinates of #C#,
#m_(AC)=3/2=(y-1)/(x-1)#,
#=> 3x-2y-1=0 ----- Eq(1)#
similarly, #m_(BC)=1/2=(y-4)/(x+1)#,
#=> x-2y+9=0 ----- Eq(2)#
#Eq(1)-Eq(2), => 2x-10=0#,
#=> x=5#, substituting #x=5# in Eq(1) or Eq(2), we get,
#y=7#,
#=># coordinates of #C=(5,7)#

Related questions
Impact of this question
1153 views around the world
You can reuse this answer
Creative Commons License