Question

Which explanation justifies how the area of a sector of a circle is derived?

Answer 1

I would go with the first one

Explanation:

AS an example:

Suppose you had a circle of diameter 10 inches then the radius
(`1/2` of the diameter) would be 5 inches

Suppose your sector was such that it was `20^0` at the centre of the circle.

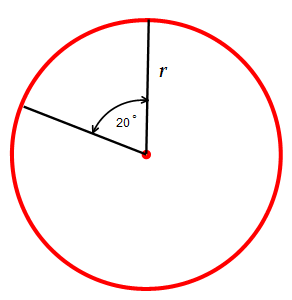
Area of the whole circle `->pir^2 =pixx5^2 = 25pi" inches"^2`

There are `360^o` in a circle so as a fraction of the whole circle the sector is `20/360`

Thus the area of the sector is `20/360xx25pi = 25/18 pi" inches"^2`
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
The answer is in fractional form and also uses `pi` as this is an exact value. Convert it to decimal and it becomes approximate.

`4.3633231299....` is an approximation of `25/18pi`

Rounding this to , say, 4.36 is even further away from `25/18 pi`