Question

Question #830d3

Answer 1

The information on the increase and decrease intervals of a differentiable function are given by the first derivative, while the information about its concavity direction are given by the second derivative (if the function is twice differentiable).

In this case `f:[0,2 pi] to mathbb{R}` such that `f(x)=7 sin x +7 cos x`. This function is a composition of twice differentiable functions, so it's twice differentiable.

The first derivative is `f'(x)=7 cos x - 7 sin x`.
Let's find the values for which `f'(x)>0`:
`7 cos x - 7 sin x >0`
`cos x >sin x`
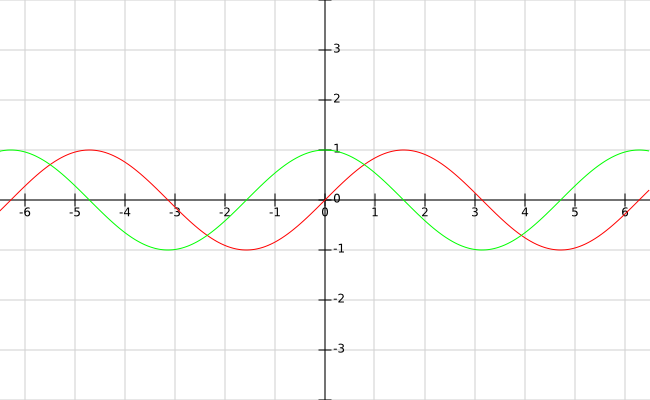
Let's look at the graphs of the two functions (`cos x` is green, `sin x` is red)

In `[0,2 pi]` they intersect when `cos x = sin x hArr tan x=1 hArr x=pi /4 + k pi` where `k=0,1`. So `cos x >sin x hArr x in (0,pi/4) cup (5/4 pi, 2 pi)` and `cos x < sin x hArr x in (pi/4,5/4 pi)`.
By the increase/decrease test and the first derivative test :

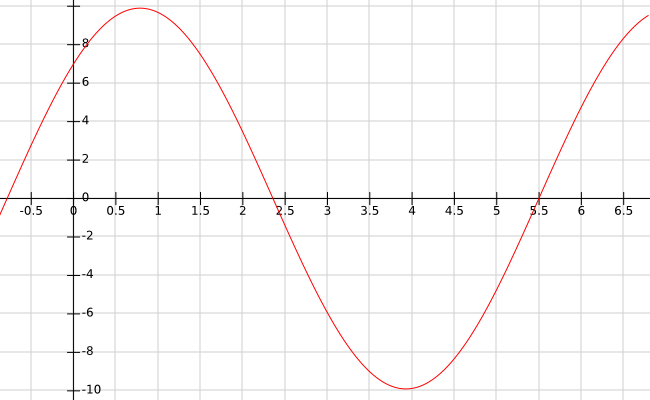
• `f` increases on `(0,pi/4) cup (5/4 pi, 2 pi)`;
• `f` decreases on `(pi/4,5/4 pi)`;
• the local maxima of `f` are at `x=pi/4` and `x=2 pi`; their values are respectively `f(pi/4)=7 sqrt(2)` and `f(2pi)=7`;
• the local minima of `f` are at `x=0` and `x=5/4 pi`; their values are respectively `f(0)=7` and `f(5/4 pi)=-7 sqrt(2)`;

The second derivative is `f''(x)=-7 sin x-7cos x`.
Let's find the values for which `f''(x)>0`:
`-7 sin x - 7 cos x >0`
`cos x < - sin x`
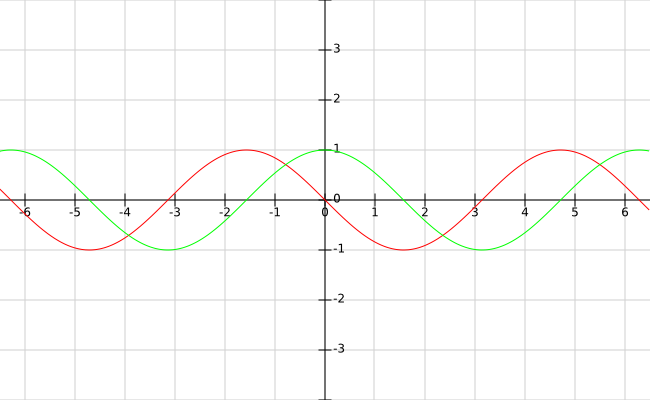
As before, we can plot the two functions (`cos x` is green, `-sin x` is red)

In `[0,2 pi]` they intersect when `cos x = - sin x hArr tan x=-1 hArr x=3 /4 pi + k pi` where `k=0,1`. So `cos x < -sin x hArr x in (3/4 pi, 7/4 pi)` and `cos x > -sin x hArr x in (0,3/4 pi) cup (7/4 pi, 2 pi)`.
By the concavity theorem:

• `f` is concave upward on `(3/4 pi, 7/4 pi)`;
• `f` is concave downward on `(0,3/4 pi) cup (7/4 pi,2pi)`;

The graph of this function is plotted in the following picture (warning: plot realized using different scaling factors on the two cartesian axes)