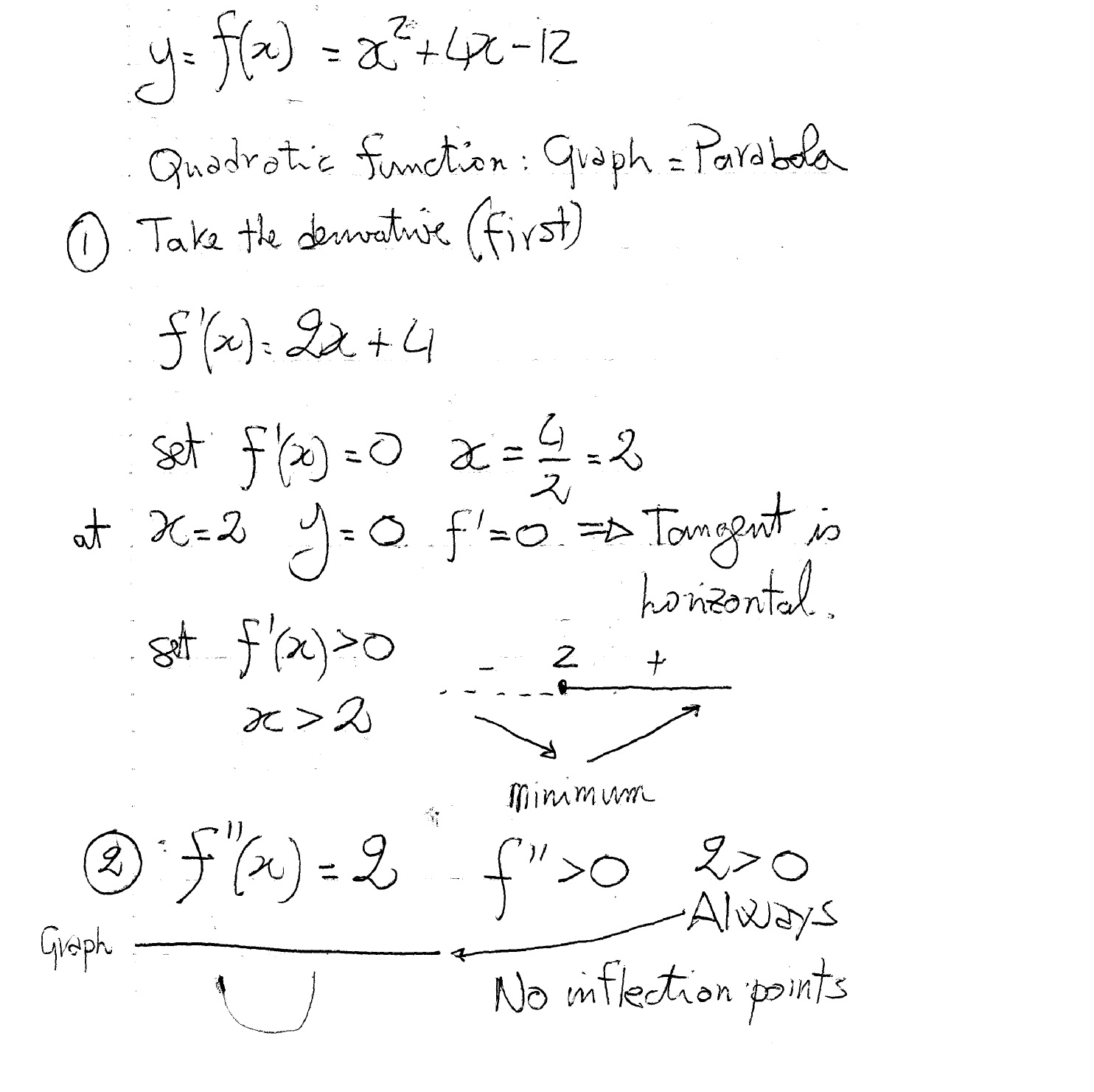
How do you find the critical points for #f(x)= x^2 + 4x - 12#?
1 Answer
Mar 26, 2015
You have to find, basically, where your function "changes" orientation. This can be a point of maximum or minimum or a point of inflection.
If you study the first derivative of your function you can see when your function has an horizontal tangent (i.e. where the derivative is equal to zero) meaning that there you have a minimum or maximum. To decide whether it is a maximum or minimum you set the derivative bigger than zero.
To detect inflection points you do the same but with the second derivative.