Question

Show that the roots of the equation `16x^4 - 20x^2 + 5= 0` are `cos((kx)/10)`for `k = 1,3,7` and `9` And Deduce that `cos^2(pi/10)cos^2((3pi)/10) = 5/16`?

Answer 1

Antoine, I think that your roots are without `x` but with `pi` instead:
To solve your equation substitute `x^2=t` to reduce the degree but remember at the end to go back to x:

Your roots corresponds to `cos(kpi/10)` with `k=1,3,7,9`

and if you have:

Answer 2

`f(x) = 16x^4-20x^2+5`

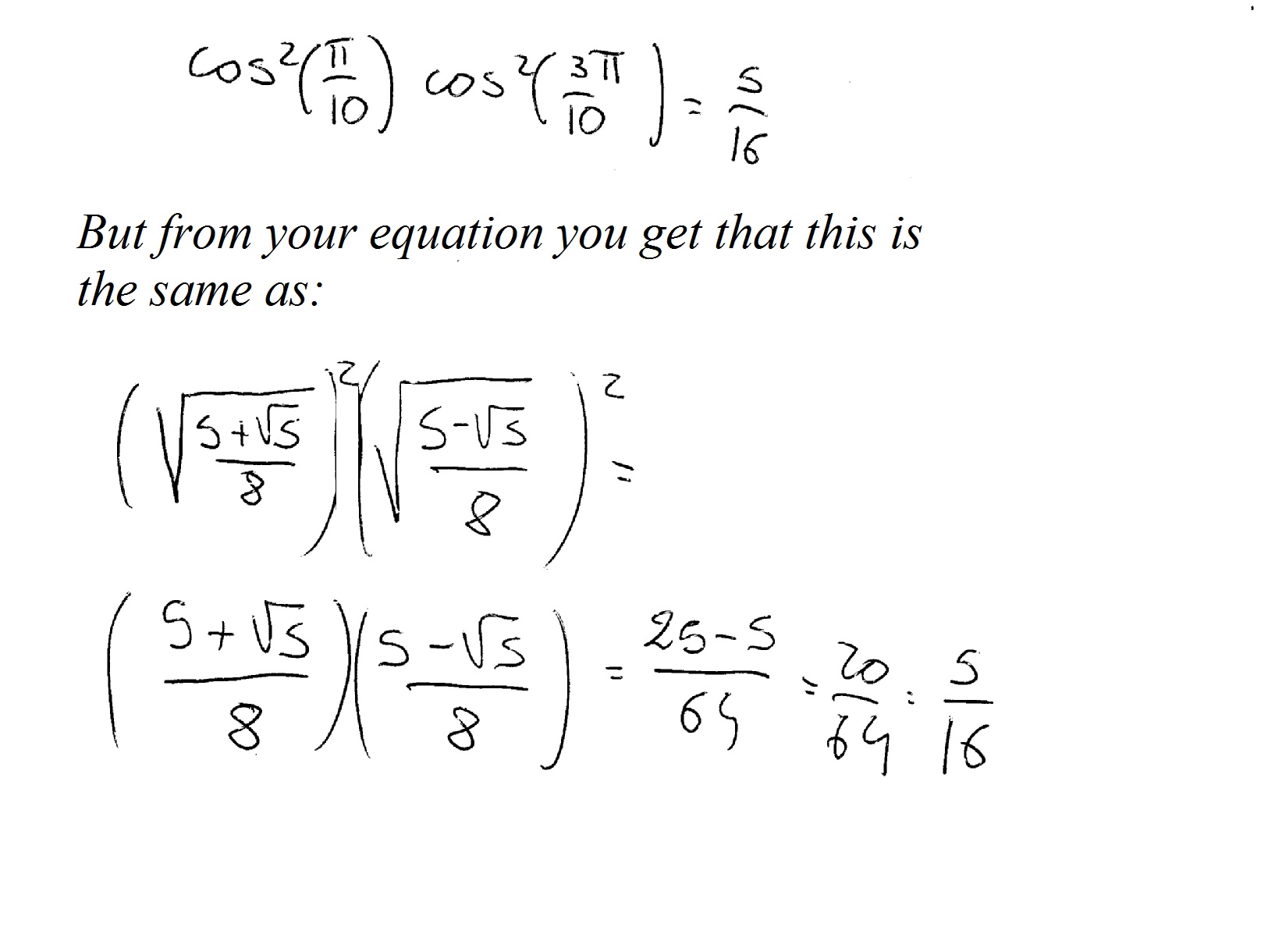
Has real zeroes, because
`-b+-sqrt(b^2-4ac) = -(-20)+-sqrt((20)^2-4(16)(5))`
`=20+-sqrt(20^2-16*20) = 20+-sqrt(4*20)` is positive.

For the full solution, see Gio's answer.)

`f` is an even function, so its four (real) zeroes occur in opposite pairs: `p_1, p_2` (the positive zeros) and `-p_1, -p_2`.

The factor theorem gives us:
`f(x)= 16(x-p_1)(x+p_1)(x-p_2)(x+p_2)`

The constant for this product is clearly `16(p_1)^2 (p_2)^2` which must be equal to `5`.

Therefore, `(p_1)^2 (p_2)^2=5/16`.

For the first part

Expand `cos(5t)` or use the multiple angle formula to get:

`cos(5t) = 16cos^4t-20cos^2t+5`

Then with `t= pi/ 10` we get:

`16cos^4 (pi/10) -20cos^2 (pi/10)+5 = cos(5( pi/10)) =cos (pi/2) = 0`.

In fact, with `t=(k pi)/ 10` we get:
`16cos^4 ((k pi)/ 10) -20cos^2 ((k pi)/ 10)+5 = cos(5((k pi)/ 10)) =cos ((k pi)/2) = 0` whenever `k` is odd.