Question

Question #b43b8

Answer 1

I found `28m/s` directed downwards.(but check my maths)

Explanation:

When the object comes back (passing through the releasing point again) it'll have a velocity of `13.3m/s` but directed downwards (neglecting anty air resistance)!
So we have:

Answer 2

`v = -"27.5 m/s"`

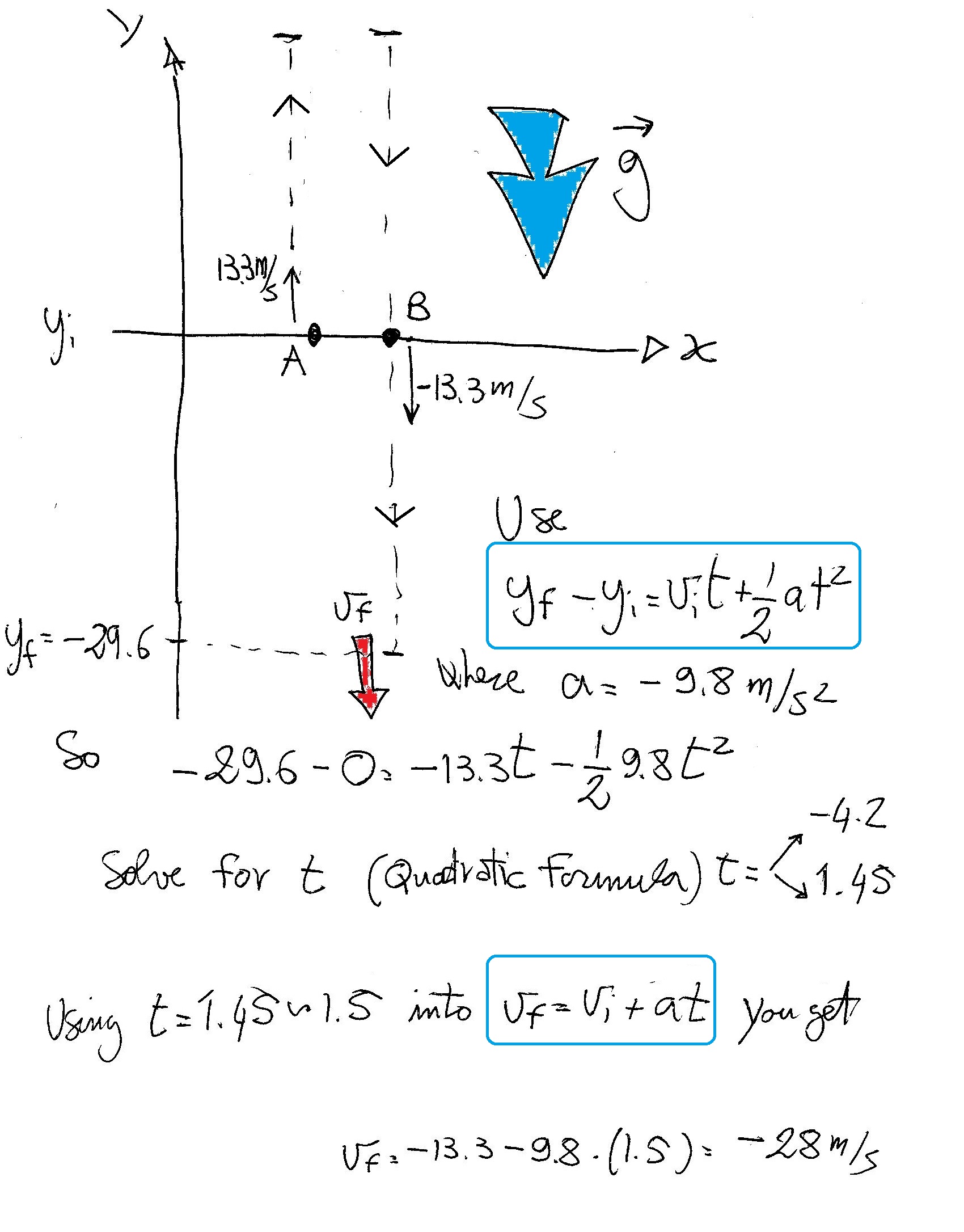
Explanation:

So, you know that an object is being thrown straight upwards from an unknown height `h` above the ground with an initial velocity of `"13.3 m/s"`.

At meximum height, the velocity of the object will be equal to zero, which means that you can say

`underbrace(v_"top"^2)_(color(blue)(=0)) = v_0^2 - 2 * g * h_1`

The distance the object travels before reaching maximum height will thus be

`h_1 = v_0^2/(2 * g) = (13.3^2 "m"^2color(red)(cancel(color(black)(2))) color(red)(cancel(color(black)("s"^(-2)))))/(2 * 9.8color(red)(cancel(color(black)("m"))) color(red)(cancel(color(black)("s"^(-2))))) = "9.03 m"`

Now, you know that you must determine the object's velocity when it is located `"29.6 m"` below the point of its release.

This is equivalent to saying that you must find its velocity after the fell for a total of

`"9.03 m" + "29.6 m" = "38.63 m"`

So, if the object is free falling for a distance of `"38.63 m"`, then you can say that

`v_"x"^2 = underbrace(v_"top"^2)_(color(blue)(=0)) + 2 * g * "38.63 m"`

The velocity of the object will thus be

`v_"x" = sqrt( (2 * 38.63"m" * 9.8"ms"^(-2))) = "27.5 m/s"`

If we take the upwards direction to be the positive direction, then the velocity of the object will be

`v_"x" = color(green)(-"27.5 m/s")`