How do you find the volume of the region enclosed by the curves y=x, y=-x, and x=1 rotated about the y axis?
2 Answers
Oct 10, 2015
If rotating about the y-axis, the shell method is your best bet to a quick solution.
Explanation:
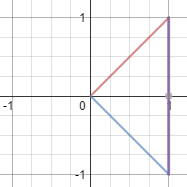
hope that helped
Oct 10, 2015
Look at the big picture then break it apart into extrusions.
Explanation:
https://www.desmos.com/calculator/zem7gewjxm
I see it like a (Cylinder-2Cone) instead of a funky shape. So if we find the volume of the cylinder we get
Now we have 2 Cones which are


