Question

The width of a rectangle is fixed at 28 cm. What lengths will make the perimeter greater than 72 cm?

Answer 1

`l > "8 cm"`

Explanation:

Start by writing down the formula for the perimeter of a rectangle

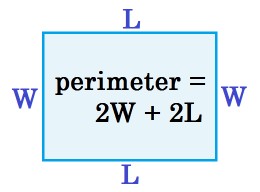
`"perimeter" = P = 2 xx (l + w)" "`, where

`l` - the length of the rectangle;
`w` - its width.

In your case, you know that the width of the rectangle is set at `"28 cm"`. In order to find which lengths would make the perimeter greater than `"72 cm"`, determine what exact length will make the perimeter exactly `"72 cm"`.

`P = 2 xx (l + 28) = 72`

`l + 28 = 72/2`

`l = 36 - 28 = "8 cm"`

This means that for any length that exceeds `"8 cm"`, the perimeter of the rectangle will be greater than `"72 cm"`.

`2 x (l + 28) > 72`

`l + 28 > 36`

`l > "8 cm"`

SIDE NOTE Don't be confused by the fact that the length turned out to be "shorter" than the width, that happens in some cases.