Question

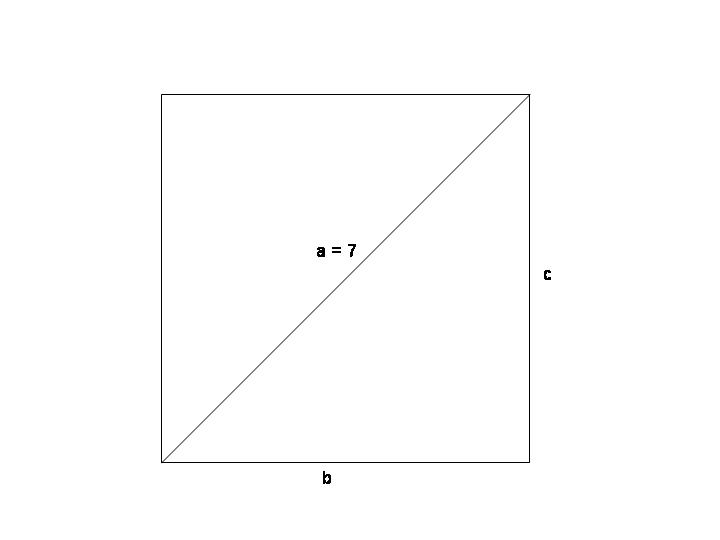
The diagonals of a square each measure 7 feet. How do you find the area of the square?

Answer 1

4.95 feet

Explanation:

You must use trigonometry.
Since it is a square, and each angle in a square is 90 degrees, the two angles of the triangle the diagonal makes is half of that, 45.

`sintheta =`(opposite)/hypoteneus
`sin45 = o/7`
`7sin45= o`
`o = 4.94974746831`

Answer 2

You can use the Pythagorean Theorem

`A=24.5` square feet

Explanation:

The Pythagorean Theorem says that in a square triangle the square of the hypotenuse (a) is equal to the square of one leg (b) plus the square of the other lag (c):

`a^2=b^2+c^2`

The diagonal of a square form a square triangle with two of the sides (see figure below) and (b) is equal to (c). Therefore, the equation can be written as below:

`a^2=b^2+b^2`

`7^2=b^2+b^2`

`49=2b^2`

`b^2=49/2`

`b=sqrt(49/2)` feet (side of the square)

The area is the product of two sides

`A=sqrt(49/2)xxsqrt(49/2)=49/2=24.5` square feet