Question

How do you solve the AP Calculus AB 2013 Free Response question #2? http://media.collegeboard.com/digitalServices/pdf/ap/apcentral/ap13_frq_calculus_ab.pdf

Answer 1

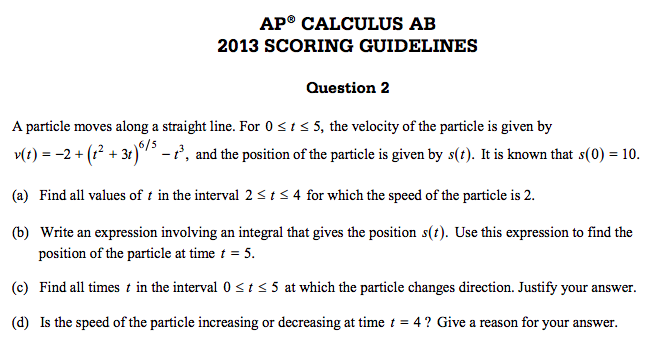
(a) The first thing you do is that you set `abs(v(t)) = 2`. It should look like this: `2=abs(-2+(t^2+3t)^(6/5)-t^3`. Then you change the output to 2 and -2 to get rid of the absolute value. You then move the twos to one side so you get `0=(t^2+3t)^(6/5)-t^3` and `0=(t^2+3t)^(6/5)-t^3-4`. You then insert them into a calculator and find the zeroes between 2 and 4. Your answer should be t=3.128, 3.473 .

(b) To find the expression you need to know your bounds. Since this is a time function, you know that your lower bound is 0, and since it is an expression for any part of the function your upper bound is t. You have to add 10, because `s(0)=10`. Your integral should look like `s(t)=10+int_0^t(-2+(t^2+3t)^(6/5)-t^3)`. To find t=5, you have to do s(5)-s(0). You can do this by using fnInt which is math 9 on a ti 84. You put in 5 as your upper bound 0 as your lower bound and put the equation in y1. To account for the 10 you add the 10 after you solve the equation. This should give you -9.207.

(c) To do this you have to find all the times that v(t) changes sign. You can do this by looking at a graph on your calculator, and looking between 0 and 5. The values should be t=.536,3.318

(d) To find the acceleration, you have to find the derivative of the velocity function. You do this by using the power rule and the chain rule. The derivative should be `v’(t)=(6/5)(t^2+3t)^(⅕)*(2t+3)-3t^2`. You then plug in a four into v’(t) and this gives you -22.296. You then have to do v(4) and this gives you -11.476. Since both velocity and acceleration have the same sign at t=4, the speed is increasing.

Answer 2

Here is a link to the answer here on Socratic http://socratic.org/questions/how-do-you-solve-the-ap-calculus-ab-2013-free-response-question-2-http-media-col