Question

How do you use the shell method to set up and evaluate the integral that gives the volume of the solid generated by revolving the plane region `y=x+2` and `y=x^2` rotated about the x axis?

Answer 1

See the explanation section, below.

Explanation:

Graph the region, including the points of intersection, `(-1,1)` and `(2,4)`.

In order to use shells, we must take our representative slices parallel to the axis of rotation. So the thickness of each shell will be `dy`.

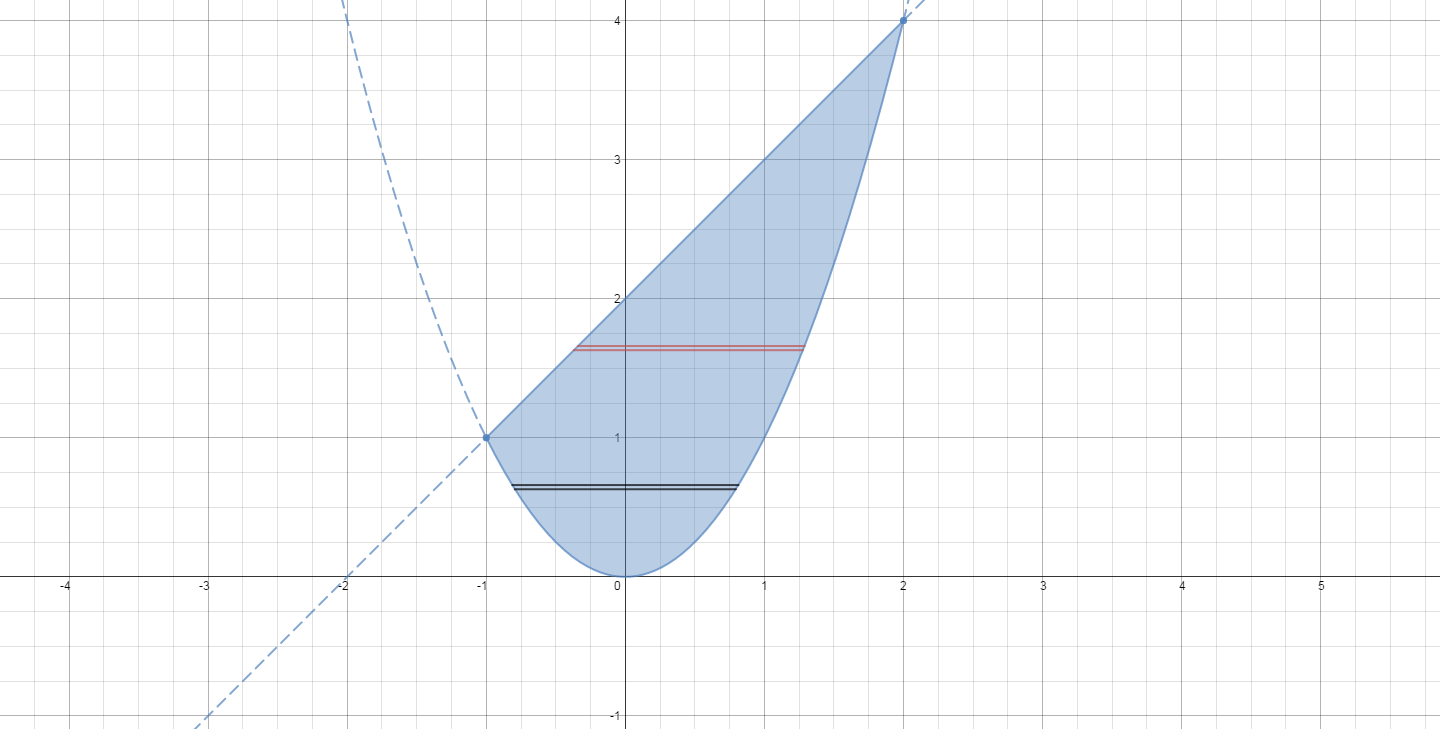
The thickness of each shell will be `dy` and we will be integrating with respect to `y`. So, we need to express the boundaries as function of `y`:

`x=y-2` and `x= +- sqrty`

And the limits of integration are `0` to `4`

The volume of the representative shell is `2pi*"radius"*"height"*dy`

The radius of each shell will be `y`

The "height" of each cylindrical shell will be the distance between the `x` values. This will be the `x` on the right (`x_"right"`) minus the `x` on the left (`x_"left"`).

Notice in the picture, that as `y` goes from `0` to `4`, the values of `x_"right"` and `x_"left"` change.

From `y=0` to `y=1` , we have:
`"height" = x_"right" - x_"left" = sqrty - (-sqrty) = 2sqrty = (8pi)/5`

So we need

`int_0^1 2 pi y (2sqrty) dy = 4pi int_0^1 y^(3/2) dy`

From `y=1` to `y=4` , we have:
`"height" = x_"right" - x_"left" = sqrty - (y-2)`

So we need

`int_1^4 2 pi y (sqrty-y+2) dy = 2pi int_1^4 (y^(3/2)-y^2+2y) dy = (64pi)/5`

The total volume is `(8pi)/5 + (84pi)/5 = 72/5 pi`