Question

What are the critical points of `f(x,y) = e^(x^3) + y^3 - e^(xy)`?

Answer 1

The critical points are `(x,y)=(0,0)` and `(x,y) approx (0.3705, 0.3768)`. The first one is a saddle point and the second one is a local minimum.

Explanation:

The partial derivatives are:

`(partial f)/(partial x)=3x^2e^(x^3)-ye^(xy)` and `(partial f)/(partial y)=3y^2-xe^(xy)`.

If you set these both equal to 0, the resulting system of equations clearly has `(x,y)=(0,0)` as a solution. You must use a calculator or software to approximate the other solution, and it turns out to be `(x,y) approx (0.3705, 0.3768)`.

The first one is a saddle point and the second one is a local minimum.

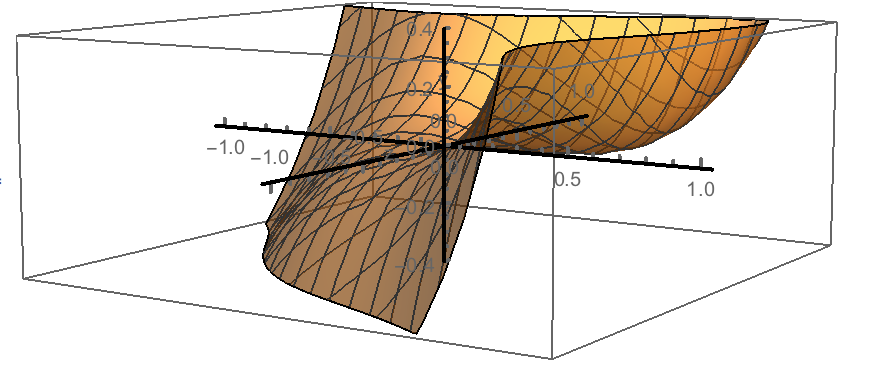
Below is a 3-dimensional picture of the graph of this function. The positive `x`-axis points to the right and the positive `y`-axis points into the screen.