Question

A block weighing `6 kg` is on a plane with an incline of `(5pi)/6` and friction coefficient of `4/5`. How much force, if any, is necessary to keep the block from sliding down?

Answer 1

0.8N up the slope.

Explanation:

Start by setting your problem up in a diagram like so:

(`g` is the acceleration due to gravity, value `=9.81ms^-2`)
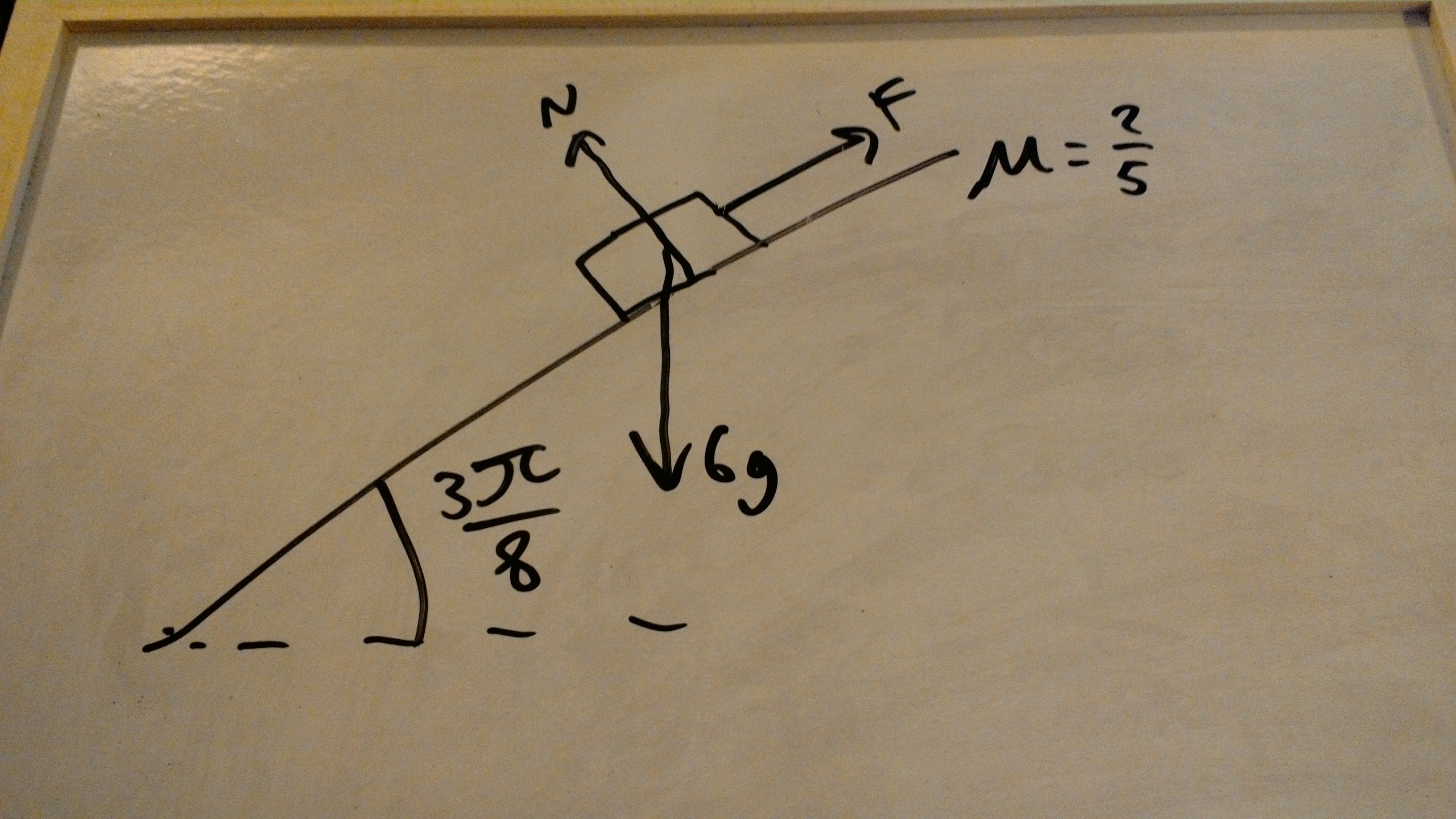
Then think about which formulae and physics concepts you will need to solve the problem.

We have the idea of equilibrium. We want the forces to balance so that the block doesn't move. So any resultant force should be zero.

We are mostly concerned with the forces parallel to the slope. Let's calculate the friction.

`F=muR`

Where `F` is friction, `mu` is the coefficient of friction, and `R` is the normal reaction force.

So from my diagram we get the result:
`F=2/5N`

To find `N` we resolve forces perpendicular to the slope:

`N=6gcos((3pi)/8)`

So now we have

`F=2/5*6gcos((3pi)/8)`

Now we will resolve parallel to the slope, remembering that we want the resultant force to equal zero, so we will need to introduce an additional force to balance it out.
I will call it `T`, and define it as acting up the slop, in the same direction as `F`.

`F+T-6gsin((3pi)/8)=0`

`2/5*6gcos((3pi)/8)+T-6gsin((3pi)/8)=0`

`T=6gsin((3pi)/8)-2/5*6gcos((3pi)/8)`

`T=6g(sin((3pi)/8)-2/5cos((3pi)/8))`

Numerical answer, `T=0.770806....`
`T=0.8N` (1 s.f.)