Question

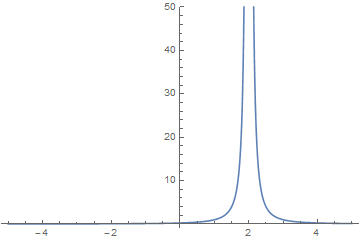
For what values of x, if any, does `f(x) = 1/(x^2-4x+4)` have vertical asymptotes?

Answer 1

`x=2`.

Explanation:

To find the vertical asymptotes we have to find for which values the denominator is equal to 0. Simply set the polynomial on the denominator to 0 then solve for `x`:

`x^2-4x+4=0`
`(x-2)(x-2)=0`
`therefore x = 2`

Thus there is one vertical asymptote at `x=2`.

Here is a graph of the function, the vertical asymptote is clearly indicated by a steep incline towards `x=2`.