Question

In a calorimeter, 1.0 kg of ice melts at 0°C. The enthalpy of fusion of the ice is 334 J/g. How much heat was absorbed?

Answer 1

`"330 kJ"`

Explanation:

A given substance's enthalpy of fusion, `DeltaH_"fus"`, tells you how much heat is needed in order to convert one gram of that substance from solid at its melting point to liquid at its melting point.

It's important to remember that phase changes take place at constant temperature, which is why you'll sometimes see the enthalpy of fusion being referred to as the latent heat of fusion.

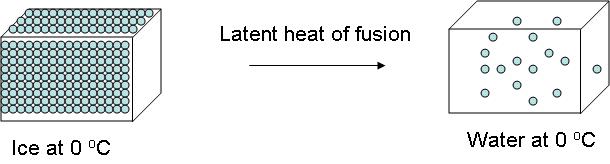
So, ice has an enthalpy of fusion equal to `"334 J g"^(-1)`. This means that in order to convert `"1 g"` of solid ice at `0^@"C"` to `"1 g"` of liquid water at `0^@"C"`, you need to provide it with `"334 J"` of heat.

To determine how much heat is needed to convert `"1.0 kg"` of solid ice to liquid water, both at `0^@"C"`, you can use the enthalpy of fusion as a conversion factor.

First, convert the mass of the sample to grams by using the conversion factor

`"1 kg" = 10^3"g"`

This will get you

`1.0 color(red)(cancel(color(black)("kg"))) * (10^3"g")/(1color(red)(cancel(color(black)("kg")))) = 1.0 * 10^3"g"`

Use the mass of the sample and the enthalpy of fusion of ice to find

`1.0 * 10^3color(red)(cancel(color(black)("g"))) * overbrace("334 J"/(1color(red)(cancel(color(black)("g")))))^(color(purple)(DeltaH_"fus")) = 334 * 10^3"J"`

You need to round this off to two sig figs, the number of sig figs you have for the sample of ice. You can also express the result in kilojoules by using the conversion factor

`"1 kJ" = 10^3"J"`

The answer will thus be

`"heat absorbed" = color(green)(|bar(ul(color(white)(a/a)"330 kJ"color(white)(a/a)))|)`