Question

How do you find the general form of the equation of a circle with this equation in standard form `(-2-h)^2+(3-k)^2=25`?

Answer 1

Assuming that the intent of the given equation is that `(x,y)=(-2,3)` is a point on the circumference of the circle and `(h,k)` is the center
then there is no simple standard form equation.

Explanation:

The intent of this question is not completely clear (at least to me).

I have assumed that what we are given is intended to be of the generalized form:
`color(white)("XXX")(x-h)^2+(y-k)^2=r^2`
with given (sample) point for `(x,y)` and radius squared `r^2`
with the objective of finding constants for the center `(h,k)`

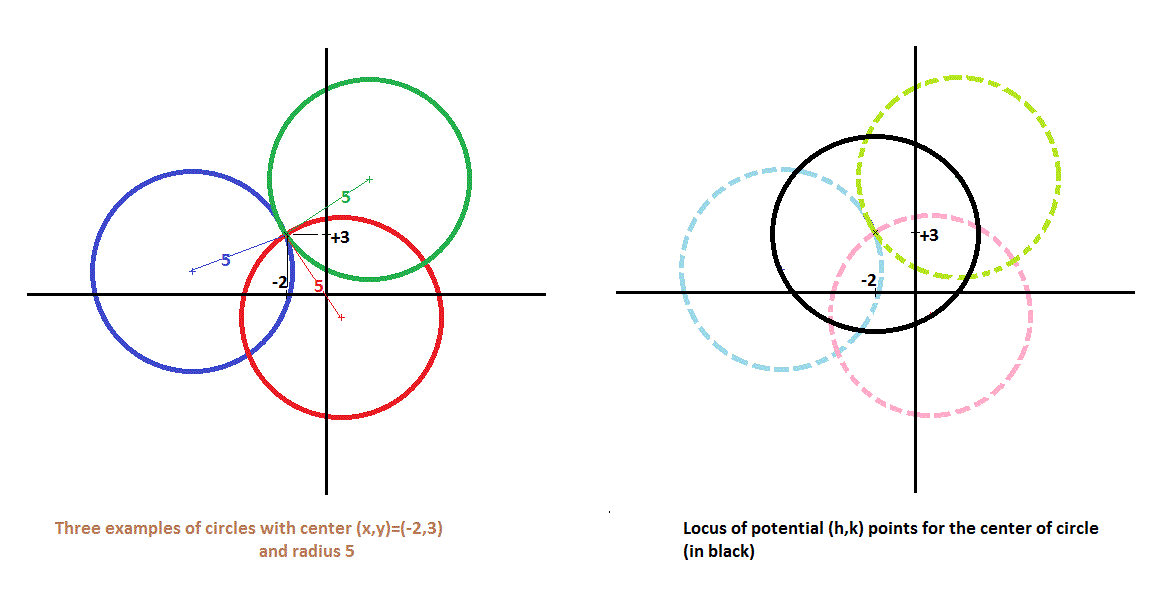
However as can been seen in the image below there are multiple (infinite number) of circles with `(x,y)=(-2,3)` as a point on the circumference and radius of `r=5` (i.e. `r^2=25`).

The collection of centers for these circles form a circle themselves at a distance of `5` from the given point `(-2,3)`