Question

An isosceles triangle has sides A, B, and C with sides B and C being equal in length. If side A goes from `(5 ,1 )` to `(3 ,2 )` and the triangle's area is `16`, what are the possible coordinates of the triangle's third corner?

Answer 1

I have taken you nearly to the end. It just a matter of algebraic manipulation.
`color(blue)("Someone else may be able to think of a simpler method!")`
`color(blue)("Perhaps utilising transformation")`

Explanation:

In geometry it always a good idea to draw a quick sketch. It helps clarify what you need to do and also shows the person marking your work that you have given thought about the solution.
`color(brown)("It also gets you extra marks")`

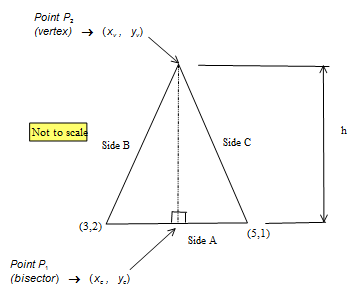
Given:
Length of side B is equal to length of side C
Coordinates of vertex AB`->(x,y)->(3,2)`
Coordinates of vertex AC`->(x,y)->(5,2)`
Area of triangle is `16color(white)(.)"units"^2`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("Method:")`

Step 1. Relate area to length of side A to determine height h
Step 2. Determine point `P_1 -> (x_c,y_c)`
Step 3. Determine gradient of line for side A

Step 4. Determine equation of line `P_1 P_2`
Step 5. Using h determine `P_2->(x_v,y_v)`

'..................................................
`color(blue)(("Step 1")`
Using Pythagoras determine length side A

`color(green)(|A| = sqrt( (5-3)^2+(2-1)^2) = sqrt(4+1)=sqrt(5))`
`color(brown)( |A|" means the magnitude of A")`

`"Area" = ("base")/2xxh`

`=>16=sqrt(5)/2xxh" "=>" "color(green)(h=32/sqrt(5))`
'................................................................
`color(blue)(("Step 2")`

Point `P_1` is the mean of the two ends of side A

`color(green)(=>P_1->(x_c,y_c) = ((5+3)/2,(2+1)/2)->(4,3/2))`
'................................................................
`color(blue)(("Step 3")`

gradient (m)`= ("change in y")/("change in x")" "` reading left to right

`color(green)(=> m= (1-2)/(5-3) = -1/2)`
'................................................................
`color(blue)(("Step 4")`
Standard form equation: `y=mx+c`

In this case the gradient is `-1/m ->-1/[-1/2] =+2`

`y=2x+c` which passes through point `P_1->(x_c,y_c)=(4,3/2)`

Substitution gives `3/2=2(4)+c" "=>" "c= 3/2-8 = -13/2`

`color(green)(=> y= 2x-13/2)`
'..............................................................
`color(blue)(("Step 5")`

`h=" distance " P_1 to P_2`

`=>h^2=(x_v-x_c)^2+(y_v-y_c)^2`

`(32/sqrt(5))^2=(x_v-4)^2+(y_v-3/2)^2`

But from step 4 `y_v=2x_v-13/2` so by substitution

`(32/sqrt(5))^2=[x_v-4]^2+[(2x_v-13/2)-3/2]^2`

'.....................................................................
`color(magenta)("I will let you take it from this point")`

`color(brown)("Solve for "x_v" then substitute in " y_v= 2x_v-13/2)`

`color(green)("Don't forget that the vertex below the base is also a possible answer!")`