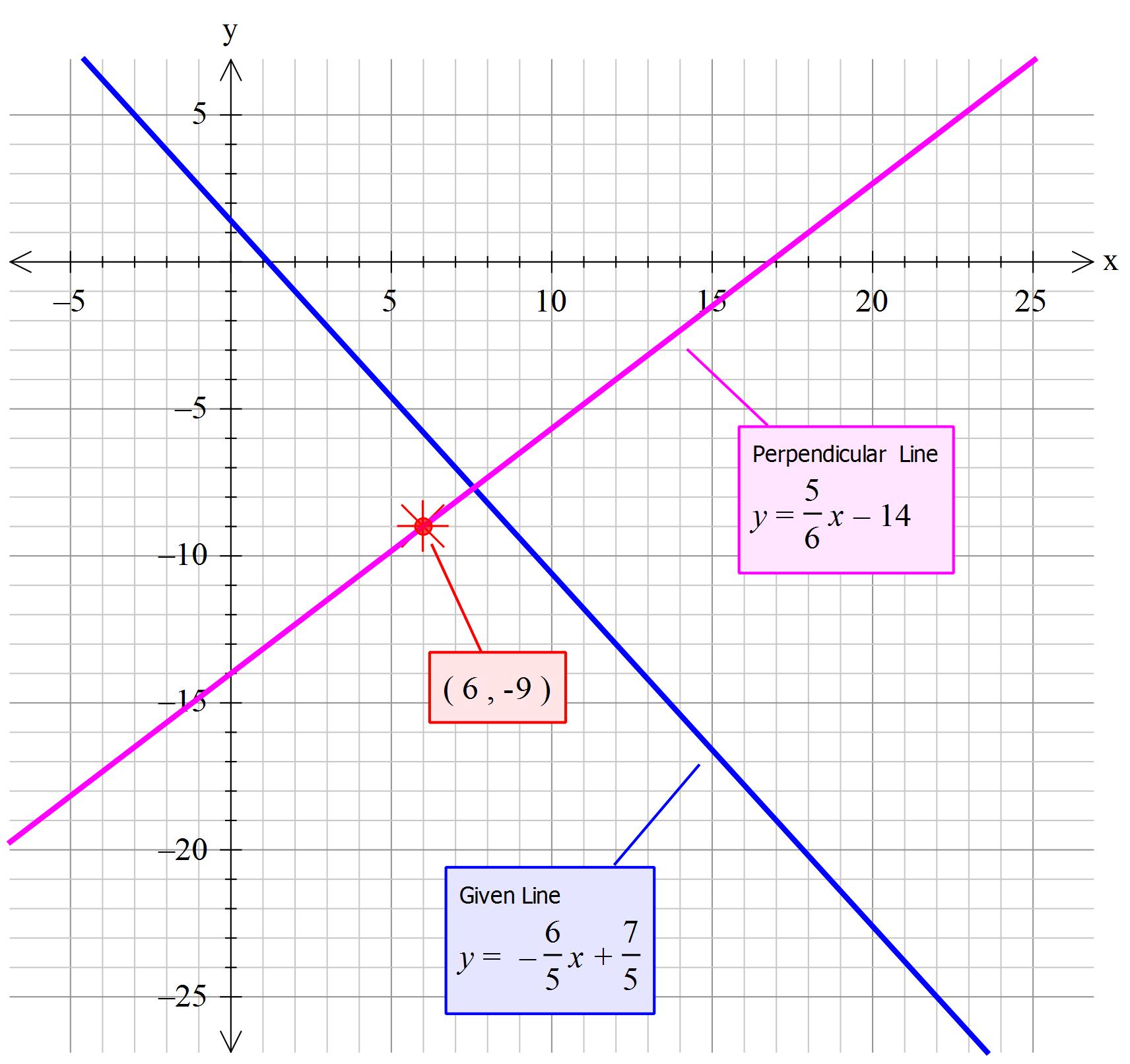
How do you write an equation of the line containing the given point and perpendicular to the given line: (6, -9); 6x+5y=7?

1 Answer
Tony B
Jul 7, 2016

#color(blue)(y=5/6x-14)#

Explanation:

Given:#" "6x+5y=7 larr" point "P_1->(x,y)=(6,-9)#

Change the equation into the standard form of #y=mx+c#

#=>y=-6/5x+7/5#............. Equation for line (1)
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

From this the gradient of the given line is:#" "-6/5#

So the gradient of a line perpendicular (normal) to this is:

#(-1)xx1/m -> (-1)xx-5/6 = +5/6#

#=>y=+5/6x+c #.....................Equation for line (2)
,................................................................................................
But the second line passes through #P_1#

#=>P_1->(x,y)=(6,-9)->-9=5/(cancel(6))(cancel(6))+c#

#=>c=-9-5=-14# giving:

#color(blue)(y=5/6x-14)#

enter image source here
'

Related questions
See all questions in Equations of Perpendicular Lines
Impact of this question
7056 views around the world
You can reuse this answer
Creative Commons License