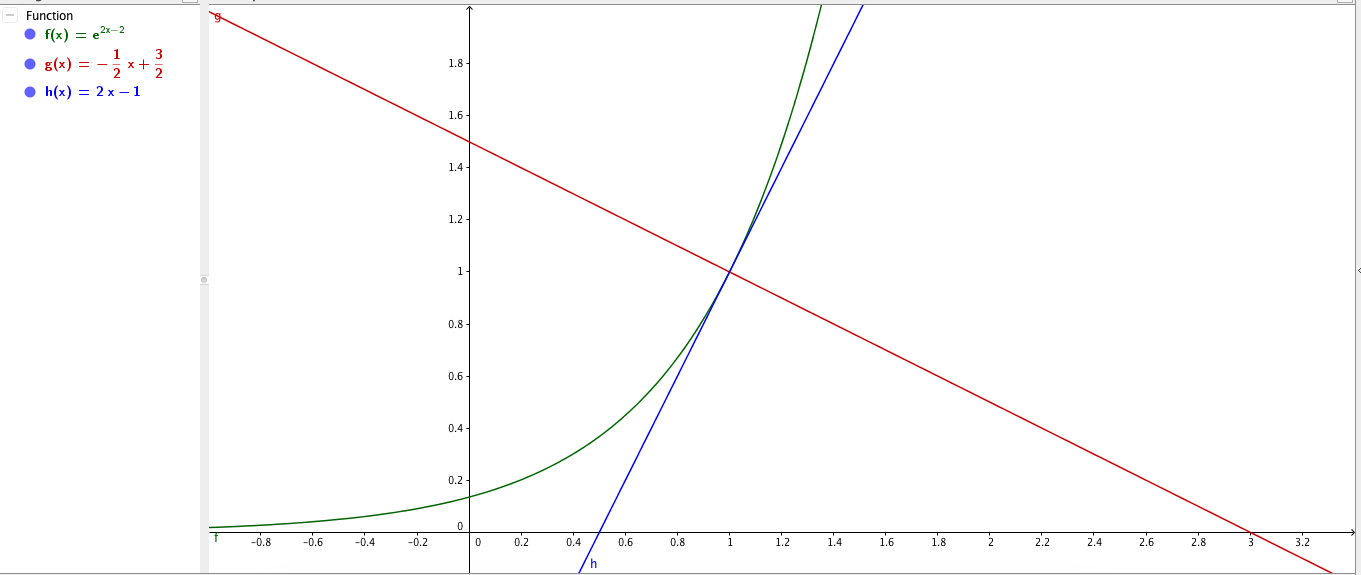
What is the equation of the line that is normal to #f(x)= e^(2x-2) # at # x= 1 #?
1 Answer
It is
Explanation:
We can first find the tangent to that curve in the point
The slope of the tangent is given by the derivative
This is the slope of the tangent line. The normal line is orthogonal to this line. The slope of the orthogonal line is
Then the normal has slope
The equation of the line is
I substitute this in the equation of the normal line
Then the equation of the normal is