Question

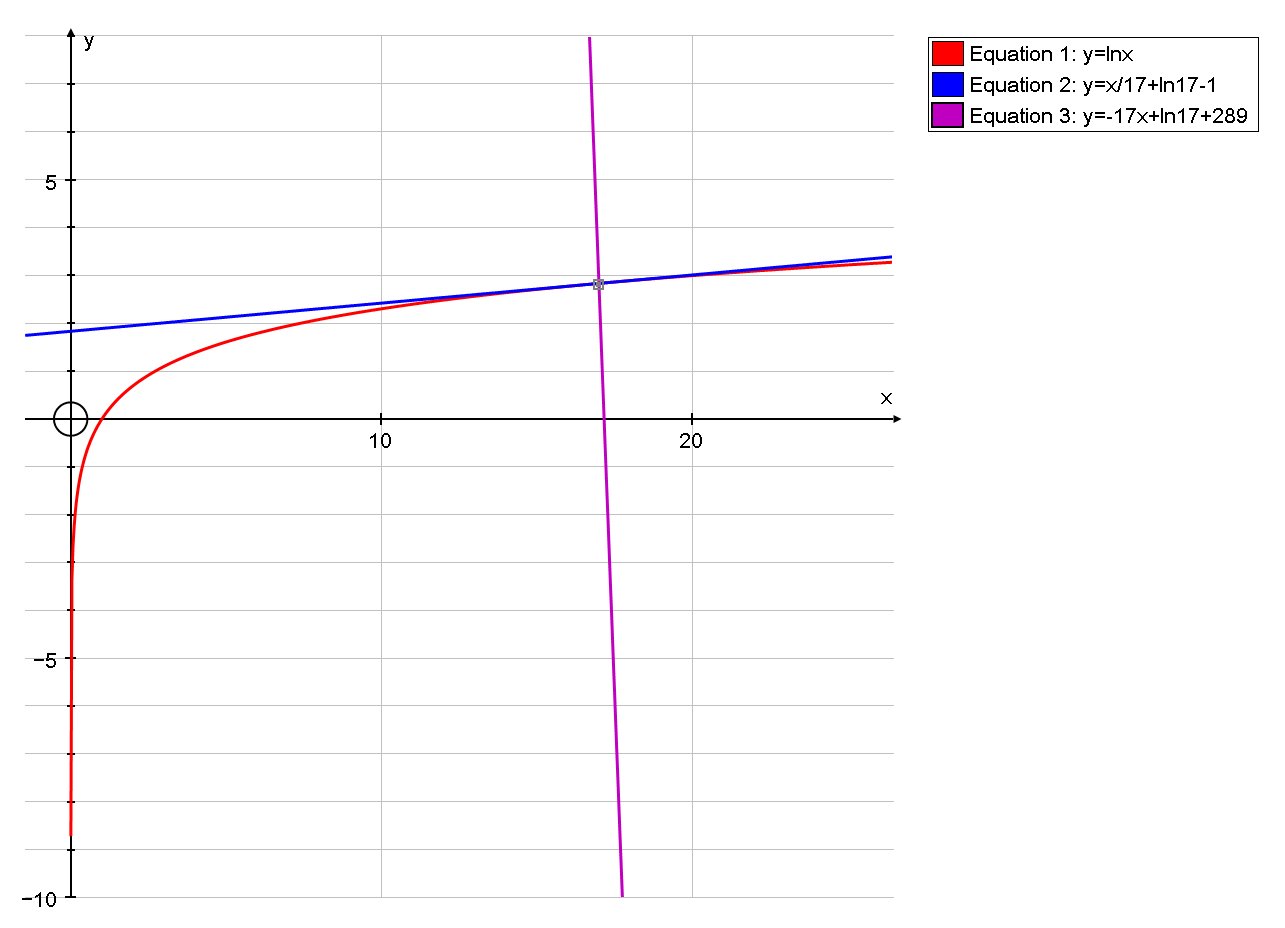
How do you find the equation of the tangent and normal line to the curve `y=lnx` at x=17?

Answer 1

Tangent Equation : `y=x/17+ln17-1`
Normal Equation : `y=-17x+ln17+289`

Explanation:

If `y=lnx` then `dy/dx=1/x`

When `x=17`
`=> y=ln17`
`=> dy/dx=1/17`

so the tangent passes through `(17,ln17)` and has gradient `m_T=1/17`

Using `y-y_1=m(x-x_1)` the equation of the tangent is:

`y-ln17=1/17(x-17)`
`:. y-ln17=x/17-1`
`:. y=x/17+ln17-1`

The normal is perpendicular to the tangent, so the product of their gradients is -1 hence normal passes through `(17,ln17)` and has gradient `m_N=-17`

so the equation of the normal is:
`y-ln17=-17(x-17)`
`:. y-ln17=-17x+289`
`:. y=-17x+ln17+289`