Question

Question #82085

Answer 1

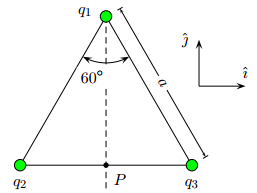
Let charges be `q_1=+10muC`, `q_2=+8muC` and `q_3=+15muC` be placed as shown in the figure above. All three vertex angles `=60^@` and all sides `a=10cm`
Now according to Coulomb's Law
`vecF_13 = k_e(q_1q_3)/r_13^ 2 hatr_13`

Inserting given values we get
`|vecF_13| = (9.0 xx 10^9)((10xx10^-6)(15xx10^-6))/(0.1)^2=135N`

Similarly
`vecF_23 = k_e(q_2q_3)/r_23^ 2 hat r_23`
From the figure we see that `hat r_23=hati`
Inserting given values we get
`|vecF_23| = (9.0 xx 10^9)((8xx10^-6)(15xx10^-6))/(0.1)^2=108N`

From the figure it is clear that angle between the two unit vectors `hatr_13 and hati` is `60^@`
As such the `x and y` components of `vecF_13` can be written as
`vecF_13=135cos60^@hati-135sin60^@hatj`
`vecF_13=67.5hati-116.9hatj`
Total force is sum of two forces
`vecF_13+vecF_23=67.5hati-116.9hatj+108hati`
`vecF_"total"=(175.5hati-116.9hatj)N`

Answer 2

`sf(211color(white)(x)N)` at an angle of `sf(56^@)` to the vertical.

Explanation:

I will suggest two methods you could use which involves finding the resultant of the two forces acting on the charge.

Here is the situation:

`sf(q_1=+10muC)`

`sf(q_2=+8muC)`

`sf(q_3=+15muC)`

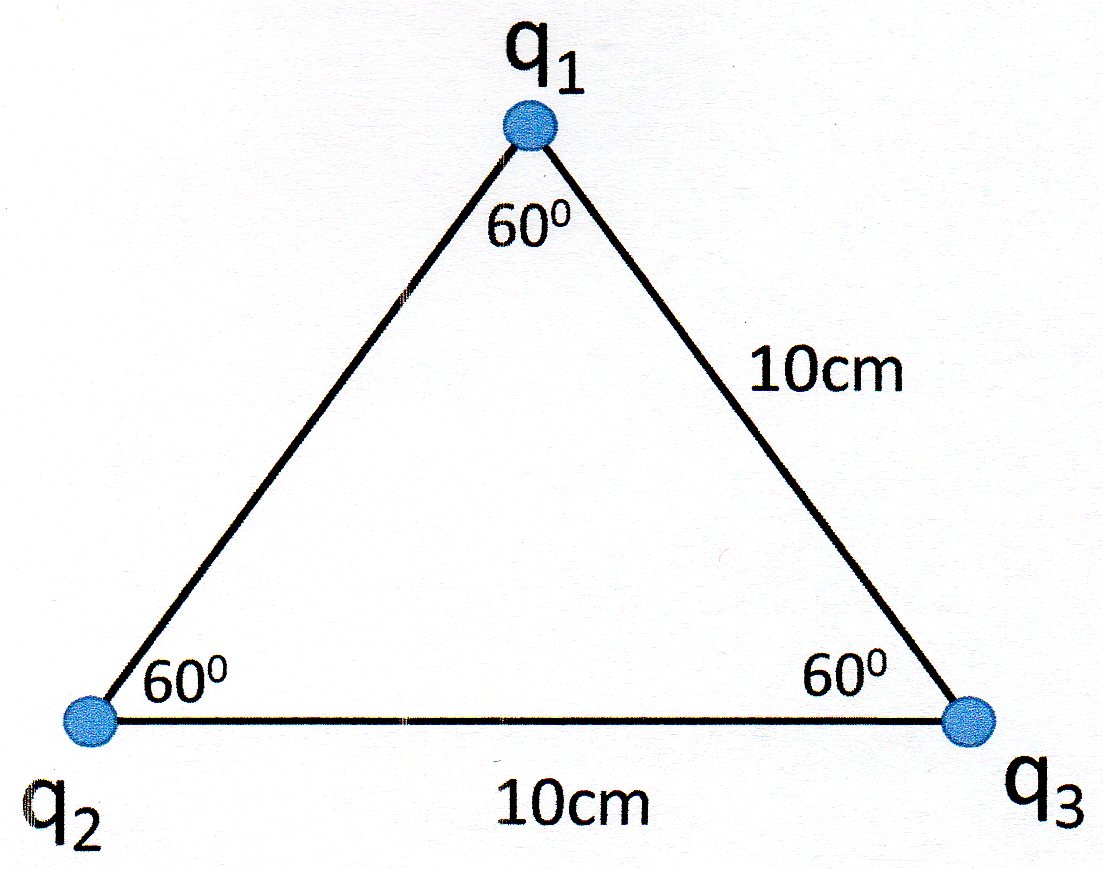
Method (1)

Here is the vector diagram showing the forces acting on `sf(q_3)`:

There is a horizontal force `sf(stackrel(rarr)(F_(23))`. There is a force `sf(stackrel(rarr)(F_(13))` at an angle of `sf(60^@)` to the horizontal.

These are indicated by the red arrows. We need to get the resultant R.

Coulombs Law gives us the force of attraction between `sf(q_2)` and `sf(q_3)`:

`sf(stackrel(rarr)(F_(23))=(kq_2q_3)/(r_(23)^2).hatr)`

`sf(k)` is a constant with the value `sf(9xx10^(9)" "N.m^(2).C^(-2))`

`:.` `sf(stackrel(rarr)(F_(23))=(kxx8xx10^(-6)xx15xx10^(-6))/(0.1^2)=120kxx10^(-10)" "N)`

By the same reasoning:

`sf(stackrel(rarr)(F_(13))=(kxx10xx10^(-6)xx15xx10^(-6))/(0.1^2)=150kxx10^(-10)" "N)`

To make the numbers easier to handle I will normalise the forces in units of `sf(K)` where `sf(K=kxx10^(-10)" "N)`

Now we have a Side Angle Side triangle. We know 2 sides and the angle between them so we can apply the cosine rule to find the unknown side.

For a triangle abc this gives us:

`sf(a^2=b^2+c^2-"2bc"CosA)`

Applying this to the vector diagram we get:

`sf(R^2=150^2+120^2-(2xx150xx120xxcos120)`

`sf(R^(2)=22500+14400-(-18000))`

`sf(R^2=54900)`

`sf(R=sqrt(54900)=234.3" "K)` units

Re-scaling:

`sf(R=234.3xx9xx10^9xx10^(-10)=color(red)(211)" "N)`

To find the angle `sf(theta)` we can apply the sine rule:

`sf(234/sin120=120/sintheta)`

`:.` `sf(sintheta=0.444)`

From which:

`sf(theta=26.3^@)`

From the diagram you can see that resultant R must be at an angle of `sf(30^@+26.3^@=56.3^@)` to the vertical.

Method (2)

We can resolve the forces into their horizontal and vertical components and find the resultant from that.

Horizontal components:

The horizontal component of `sf(stackrel(rarr)(F_(13))=sf(stackrel(rarr)(F_(13))cos60)=stackrel(rarr)(F_(13))xx0.5)`

So the total horizontal component is given by:

`sf(F_x=stackrel(rarr)(F_(23))+0.5xxstackrel(rarr)(F_(13)))`

`sf(F_x=120K+0.5x150K=195K)`

Vertical components:

`sf(stackrel(rarr)(F_(23))` does not have a vertical component so:

`sf(F_(y)=stackrel(rarr)(F_(13))cos30=150Kxx0.866=129.9K)`

Now we can apply Pythagoras:

`sf((129.9K)^2+(195K)^2=R^2)`

`sf(R^(2)=54899K^(2))`

`sf(R=234.3K" "N)`

`sf(R=234.3xx9xx10^(9)xx10^(-10)=color(red)211" "N)`

To find the angle `alpha` to the vertical:

`sf(Tanalpha=195/129.9=1.5)`

From which:

`alpha=56.3^@`

So the 2 methods are in agreement.