How should I study about moles and how they relate to things like stoichiometry?
2 Answers
You should remember that the
Explanation:
Instead of moles, we could use
You won't ever be asked to remember these masses, because you should be provided with a copy of the Periodic Table, in which molar or equivalent masses are explicitly given, in every exam in chemistry or physics you ever sit. You still have to be able to use the table competently and correctly when you determine equivalent mass.
The
So the next time you hear the term
Warning! Long answer.
You should remember that the mole is central to all stoichiometry calculations.
Explanation:
Stoichiometry is the term applied to problems that ask, "How much of
One thing we always need is a balanced equation for the reaction, because the equation tells us the ratios in which the molecules react with each other.
If we multiply the numbers of molecules by Avogadro's number,
All we have to do is to get the moles of
Usually, we have to do the calculation in three steps:
- Use the molar mass of
#bb"A"# to convert grams of#bb"A"# to moles of#bb"A"# . - Use the molar ratio of
#bb"B"# to#bb"A"# from the balanced equation to convert moles of#bb"A"# to moles of#bb"B"# . - Use the molar mass of
#bb"B"# to convert moles of#bb"B"# to grams of#bb"B"#
Here's a chart to help you navigate a stoichiometry problem.
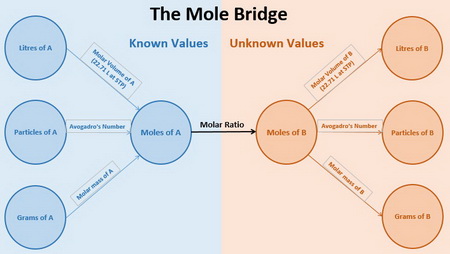
People call it the Mole Bridge, because you use moles to convert the known grams, particles, or litres of a substance
You always work from known values to the unknown (i.e. from left to right).
Here's how it works.
Sample Problem
What mass of hydrogen is needed to prepare 100.0 g of ammonia from nitrogen and hydrogen?
Solution
This is a stoichiometry problem.
I know that I will need the balanced equation and the molar masses, so I assemble all the numbers for later use.
Here, the known compound is
We start at the left of the mole bridge and work to the right.
Step 1:
Step 2:
Step 3:
You need


