A triangle has corners at #(5 , 4 )#, ( 7, 1 )#, and #( 1, 3 )#. What are the endpoints and lengths of the triangle's perpendicular bisectors?
1 Answer
End points
Explanation:
Perpendicular bisectors of a triangle, meet at a point. This point is the centre of the circumcircle of the triangle, and it is equidistant from the three vertices of the triangle. If this point is (x,y), then its distance from the points (5,4) , (7,1) and (1,3) would be
Thus,
Or,
This would give -10x+25-8y+16= -14x+49-2y+1
Or, 4x-6y=9. Similarly,
This would, on simplification as above, would give
-2x+1-6y+9= -14x+49-2y+1
Or 12x -4y=40
Solving the two linear equations in x and y would give x=
Thus one end point of all the perpendicular bisectors would be
The other end points would be the midpoints of the sides of the triangles. These would be
Or (6, 5/2), (3, 7/2)and (4,2)
The length of the perpendicular bisectors can now be calculated using the distance formula.
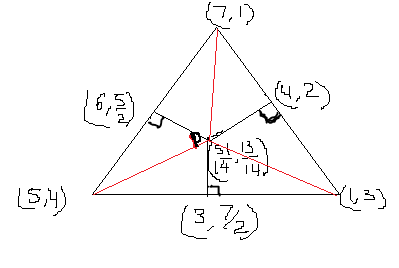
Length calculation would be a messy affair manually, hence skipped.
