Question #7477b

1 Answer
Stefan V.
Nov 24, 2016

Here's why that is the case.

Explanation:

In simple terms, displacement is the shortest distance between the start point and the finish point.

As you know, the shortest distance between two points is a straight line that connects the two points.

This means that in order to find an object's displacement, you need to examine its initial position and its final position and calculate the distance between those two points.

This implies that displacement is independent of the path taken by the object between its initial position and its final position because the shortest distance between any two points is always a straight line.

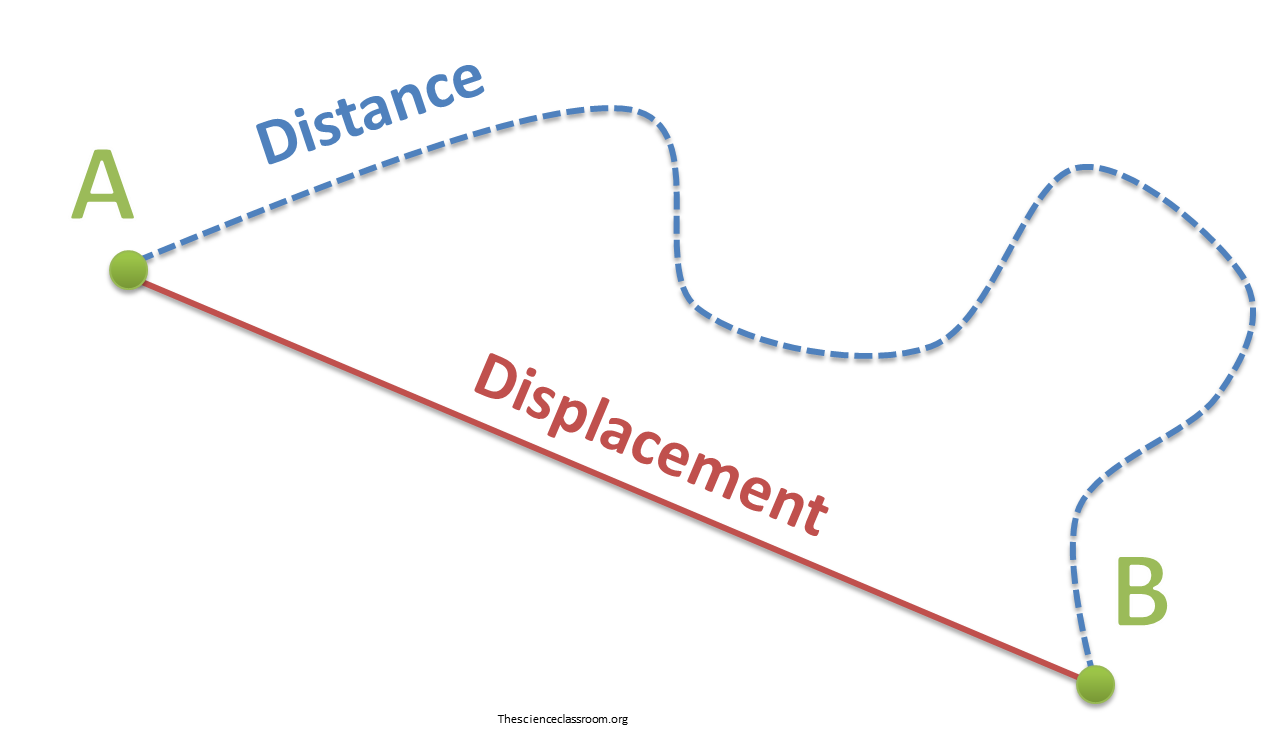
http://thescienceclassroom.org/physics/motion-in-1-d/distance-and-displacement/

Now, when you move in a circle, or in any path for which the start point is the same as the finish point, your displacement will be zero.

https://socratic.org/questions/may-you-give-an-example-where-displacement-is-zero

If the initial and final positions coincide, the displacement is equal to zero because the shortest distance between a point and itself is always zero.

Related questions
See all questions in Displacement and Velocity
Impact of this question
8129 views around the world
You can reuse this answer
Creative Commons License