A line segment is bisected by a line with the equation # 3 y - 8 x = 2 #. If one end of the line segment is at #(1 ,3 )#, where is the other end?
1 Answer
The other end is at the point:
Explanation:
When given a the equation of a line in standard form:
All lines perpendicular to the given line will be of the form:
where k is an arbitrary constant.
Write the given equation for the bisecting line in standard form:
Write the equation for all lines perpendicular to equation [3]:
The start of the bisected line segment,
Substitute 1 for x and 3 for y into equation [4] and solve for k:
The equation of the bisected line segment is:
Add 8 times equation [3] to 3 times equation [5]:
The x coordinate of the end can be found by adding the start coordinate to twice the change for the point of intersection:
Substitute
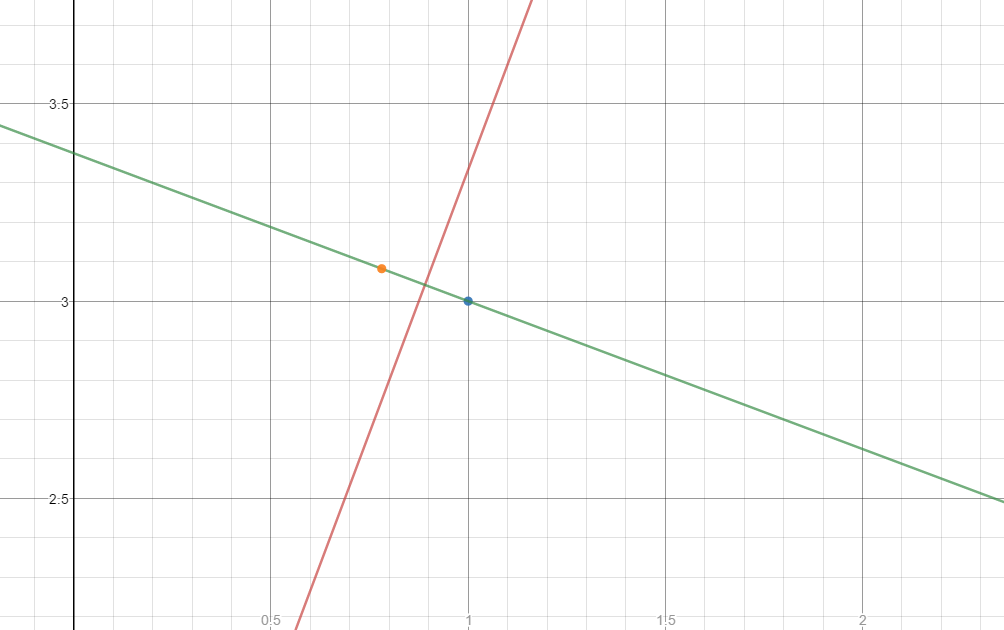
Here is a graph of, the two lines, the start point, and the end point.