How to solve the equation #z^4=ibarz# ? Where z is a complex number.
2 Answers
Explanation:
Let's think of these complex numbers in exponential forms!
So, rewriting this in terms of angles, we have:
Remembering that
Now to solve for the radius, we know that:
By taking the absolute value of both sides we have
Which we can split
But
There are only two real numbers (remember that the absolute value / radius is always real) that can satisfy this equation
# z=0,i #
# z = +-1/2sqrt(1/2(5+sqrt(5))) + 1/4(sqrt(5)-1)i#
# z = +-1/2sqrt(1/2(5-sqrt(5))) - 1/4(sqrt(5)+1)i#
Explanation:
We have:
# z^4 = i barz # ,
Where
If we use the exponential form of a complex number then we can represent the solution by;
# z= re^(itheta) \ \ \ (=rcostheta+irsintheta)#
Then the complex conjugate will be:
# z= re^(-itheta) #
Whenever dealing with complex equation such as this it is essential to remember that the complex exponential has a period of
# {re^(itheta)}^4 = i(re^(-itheta)) #
# {re^(itheta)}^4 = i(re^(i(-theta+2npi))) # where# n in NN#
(Equally we could have incorporated this periodicity into the RHS exponent, it really does not matter providing it is incorporated somewhere). Replacing
# {re^(itheta)}^4 = e^(ipi/2)(re^(i(-theta+2npi))) #
# :. r^4e^(4itheta) = re^(i(pi/2-theta+2npi)) #
Equating real and imaginary terms (or the moduli and arguments) we have:
# Re\ : \ \ r^4=r #
# Im: \ \ 4theta = pi/2-theta +2npi#
From the first equation we get:
# r^4-r = 0#
# :. r(r^3-1) = 0#
# :. r=0,1#
From the second equation we get:
# 4theta = pi/2-theta +2npi#
# :. 5theta = pi/2 +4npi/2#
# :. theta = (4n+1)pi/10#
So now we can "assemble" the solutions:
# r= 0=> z=0 #
# r= 1=> z=e^(itheta) #
And By putting
#theta = (pi)/10, pi/2, (9pi)/10, (13pi)/10, (17pi)/10, ...#
And with these values of
#n=0: => z = 1/2sqrt(1/2(5+sqrt(5))) + 1/4(sqrt(5)-1)i#
#n=1: => z = 0 + 1i#
#n=2: => z = -1/2sqrt(1/2(5+sqrt(5))) + 1/4(sqrt(5)-1)i#
#n=3: => z = -1/2sqrt(1/2(5-sqrt(5))) - 1/4(sqrt(5)+1)i#
#n=4: => z = 1/2sqrt(1/2(5-sqrt(5))) - 1/4(sqrt(5)+1)i#
After which the pattern repeats. Hence there are six solutions:
# z=0,i #
# z = +-1/2sqrt(1/2(5+sqrt(5))) + 1/4(sqrt(5)-1)i#
# z = +-1/2sqrt(1/2(5-sqrt(5))) - 1/4(sqrt(5)+1)i#
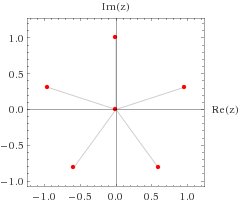
We can see these solutions on the Argand diagram: