Question

The velocity of a sailing boat in favor of the current in a river is 18km/hr and against the current, it is 6km/hr.In which direction the boat is to be driven in order to reach the other side of the river and what will be the velocity of the boat?

Answer 1

Let `v_b and v_c` respectively represent the velocity of the sailing boat in still water and velocity of current in the river.

Given that the velocity of the sailing boat in favor of the current in a river is 18km/hr and against the current, it is 6km/hr.We can write

`v_b+v_c=18........(1)`

`v_b-v_c=6........(2)`

Adding (1) and (2) we get

`2v_b=24=>v_b=12"km/hr"`

Subtracting (2) from (2) we get

`2v_c=12=>v_b=6"km/hr"`

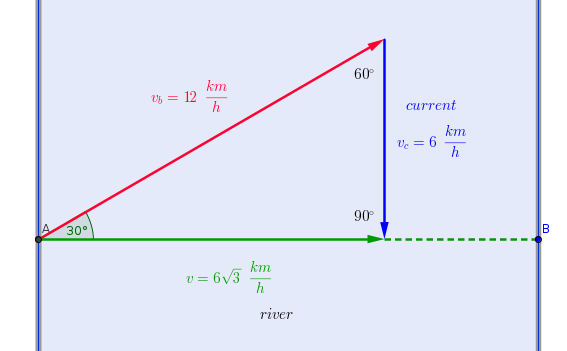
Now let us consider that `theta` be the angle against the current to be maintatined by the boat during crossing of the river to reach just opposite side of the river by sailing.

As the boat reaches just opposite point of the river, during sailing the resolved part of its velocity should balance the velocity of the current.Hence we can write

`v_bcostheta=v_c`

`=>costheta=v_c/v_b=6/12=1/2`

`=>theta=cos^-1(1/2)=60^@`

This angle is with the bank as well as with opposite direction of the current.

The other resolved part of the velocity of boat `v_bsintheta` will make it cross the river.

So this velocity

`v_bsintheta=12*sin60^@=sqrt3/2*12"km/hr"=6sqrt3"km/hr"`