I am going to assume you know something of Gauss' Law and what a Gaussian surface is.
Gauss's Law tells us that:
#int int_S mathbf E cdot d mathbf S = (sum Q_("enclosed"))/(varepsilon)#, where #varepsilon = varepsilon_r varepsilon_o#
We centre a cylindrical Gaussian surface at #rho = 0#, so that it's length runs along the cylindrical z-axis. We give our particular surface a notional height #H#.
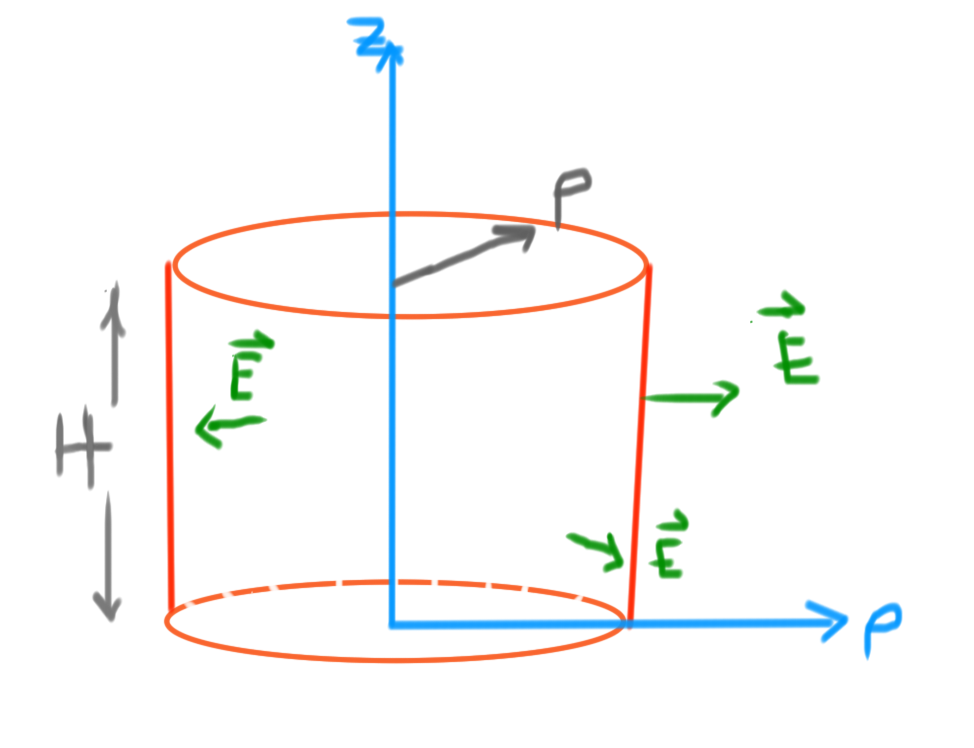
Due to symmetry, the E-field will point directly away from the surface of the cylinder at all points.
We can now use the cylindrical symmetry to say that:
#int int_S mathbf E(rho) cdot d mathbf S = E(rho) 2 pi rho H qquad triangle#
We can also use your density function, #rho_v=10/rho [pC/m^3]#, to make a volume integral:
# (sum Q_("enclosed"))/(varepsilon) = 1/epsilon int_V ρ_v(rho) \ color(blue)(dV)#
#=(10^(-11) )/epsilon int_(theta = 0)^(2 pi) \ int_(z =0)^H \ int_(rho = o)^rho 1/cancel(rho) \ color(blue) (cancel(rho) \ d rho \ d theta \ d z)#
Due to symmetry, this simplifies to:
# (sum Q_("enclosed"))/(varepsilon) = 2 pi H (10^(-11) )/epsilon int \ d rho#
# = 2 pi H (10^(-11) )/epsilon ( rho + c_1) qquad square#
Because #triangle = square#:
#E(rho) 2 pi rho H = 2 pi H (10^(-11) )/epsilon ( rho + c_1) #
#implies mathbf E(rho) = (10^(-11) )/epsilon ( 1 + c_1/rho) quad mathbf e_(rho) qquad star#
That's our electric field. We now call on this relationship between potential #V# and electric field #mathbf E = - nabla V#, which simplifies, as #rho# is the only practical independent variable, to: #mathbf E = - (partial V)/(partial rho)#.
We integrate #star# to get potential function #V(rho)#:
#V(rho) = -(10^(-11) )/epsilon ( rho + c_1 ln rho + c_2)#
We have 2 unknowns but also 2 points, ie: #V(2)= 0, V(5) =60#.
The first point tells us that:
#0 = 2 + c_1 ln 2 + c_2 implies c_2 = - 2 - c_1 ln 2 #
#implies V(rho) = (10^(-11) )/epsilon ( (rho-2) + c_1 ln (rho/2) )#
The second point tells us that:
#60 = -(10^(-11) )/epsilon ( 3 + c_1 ln (5/2) )#
#implies c_1 = ( -(60 epsilon)/ (10^(-11) ) - 3 )/(ln (5/2)) #
Rounding seems to have a material effect on the result you have posted, so what follows so this is not pretty arithmetic-wise. From here, you might want to nail some of the numbers first. This is presented in a very ugly way, but it sorta gets us an answer that is uncannily like the one you want.
From #star#:
#implies mathbf E(rho) = (10^(-11) )/epsilon ( 1 + ( (- (60 epsilon)/ (10^(-11) ) - 3 )/(ln (5/2)) )/rho) quad mathbf e_(rho) #
#= (10^(-11) )/(8.854 xx 10^(-12) cdot 3.6) ( 1 + ( ( (-60 (8.854 xx 10^(-12) cdot 3.6))/ (10^(-11) ) - 3 )/(ln (5/2)) )/rho) quad mathbf e_(rho) #
# =( 0.314 - 66.51/rho ) quad mathbf e_(rho)#


