Question

Which demand is elastic and which demand is inelastic? with the price-demand equation of 0.02x+p=60. (algebraically)

Answer 1

Demand is Relatively elastic for prices greater than `30.`
Demand is Relatively inelastic for prices less than `30.`

Explanation:

Given -

`0.02x+p=60` ------------------ (Demand function)

Demand beyond a certain price level will be elastic and price below that level will be inelastic. We have to find that price for which demand is elastic.
[Already I answer a question which is more or less like this question.
}Watch this video

Look at this diagram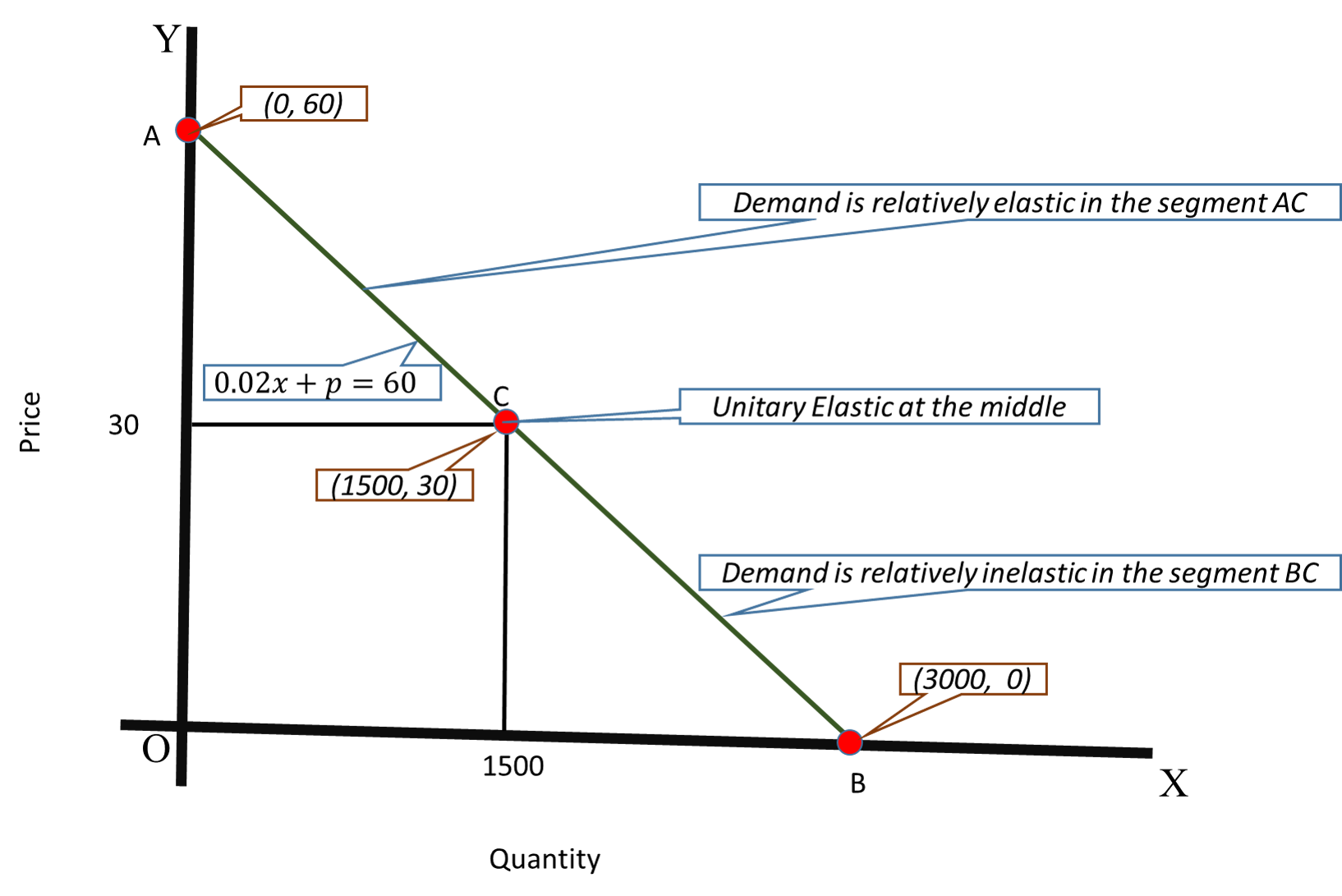

It is a linear demand curve. Find the x and y-intercepts.

At y- intercept the quantity is zero,

At `x=0; 0.02(0) + p = 60`

`p=60`
At `p=60` nothing will be demanded. Quantity is zero.
`(0, 60)` At this point the demand curve cuts the Y-axis. This is Y intercept.

At `p=0; 0.02x+0=60`

`x=60/0.02=3000`

If the price is zero, the market is willing to take 3000 units.

`(3000, 0)` At this point the curve cuts the X-axis.

Between `0, 60)` and `(3000, 0)` the straight line demand curve lies.

At the mid-point, the elasticity is 1.

Find the mid-point.

`(x, p)= (3000+0)/2 , (0+60)/2`

`(x, p) = (1500, 30)`

At the mid-point the Elasticity is unitary.

Hence -

Demand is Relatively elastic for prices greater than 30.
Demand is Relatively inelastic for prices less than 30.

Answer 2

Demand is Relatively elastic for prices greater than 30.
Demand is Relatively inelastic for prices less than 30.

Explanation:

METHOD -2

We can find the price for which elasticity is unity can also be found like this - using calculus.

The Elasticity formula in calculus is -

`ep=dx/(dp).p/x`

Rewrite the equation in terms of `x`

`0.02x=60-p`
`x=60/0.02-1/0.02p`
`x=3000-1/0.02p`

`dx/(dp) = -1/0.02`

`-1/0.02.p/x=-1`

[We want to find the price for which the elasticity is unity. Here `ep =1`. Already we ignored the minus sign, hence `-1`]

Solve it for `p`

`p=-1 xx -0.02x=0.02x`

Substitute `p=0.02x` in the demand function

`0.02x+0.02x=60`
Solve it for `x`

`x=60/0.04=1500`

Substitute `x=1500` in the demand function to find `p`

`0.02(1500)+p=60`
`30+p=60`
`p=60-30=30`

At `p=30` the elasticity of demand is unitary.

Hence -

Demand is Relatively elastic for prices greater than 30.
Demand is Relatively inelastic for prices less than 30.