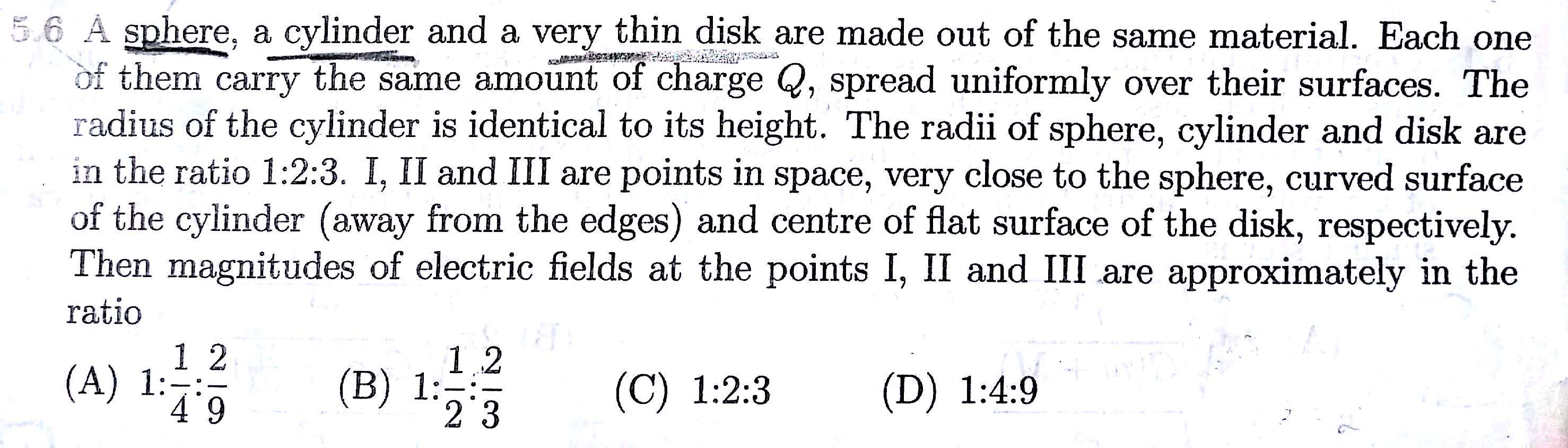
Gauss' Law #int int_A mathbf E cdot d mathbf A = Q_(enclosed)/epsilon# simplifies where there is symmetry. Basically where the #mathbf E# field always points at normal to surface, we get: #E = Q/(epsilon A)#.
So we use symmetric Gaussian surfaces.
Sphere
Here, Gaussian surface is a concentric sphere just at the surface of the real sphere.
#E_s = Q/ (epsilon \ 4 pi R^2) = Q/(epsilon pi ) 1/(4 R^2)#
Cylinder
We use a concentric cylindrical Gaussian surface, but placed near the mid-point (length-wise) of the cylinder and with height #t#.
The real cylinder's surface charge density is:
#sigma = (Q)/(2 xx pi(2R)^2 + 2 pi (2R) H) # and #H = 2R#
#= (Q)/( 16 pi R^2 )#
So our Gaussian surface encloses charge:
#2 pi (2R) t xx (Q)/( 16 pi R^2 ) = Q t/( 4 R)#.
We assume no E field through end caps of our surface, it's all going through the curved surface.
#E_c = (Q t/( 4 R))/(epsilon 2 pi (2R) t) = Q/(epsilon pi ) 1/(16 R^2)#
Disc
Here we use another concentric cylinder of small radius #rho#, but this time due to geometry we assume all the #mathbf E# field comes out via its endcaps. Our Gaussian surface contains charge #(2 xx pi rho^2)/(2 xx pi(3R)^2)Q# as it has total area normal to the #mathbf E# field of #2 pi rho^2#, ie both the end caps.
#E_d = (( rho^2)/((3R)^2)Q)/(epsilon 2 pi rho^2 ) =Q/(epsilon pi) 1/( 18 R^2 ) #
Looks like (A) to me