Question

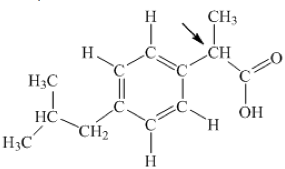
Ibuprofen has the following mass percent composition. What is the empirical formula of ibuprofen?

Answer 1

The empirical formula is `C_13H_18O_2`.

Explanation:

With all these sorts of problems, we assume a mass of `100*g` and we divide thru by the ATOMIC mass of each component:

`"Moles of carbon"=(75.69*g)/(12.011*g*mol^-1)=6.30*mol.`

`"Moles of hydrogen"=(8.80*g)/(1.00794*g*mol^-1)=8.73*mol.`

`"Moles of oxygen"=(15.51*g)/(15.999*g*mol^-1)=0.969*mol.`

We divide thru by the LOWEST molar quantity (that of oxygen) to get a trial empirical formula of `C_(6.50)H_9O`. But, by definition, the empirical formula is the SIMPLEST WHOLE NUMBER ratio defining constituent atoms in a species. So we double this trial formula to get............

`C_13H_18O_2`

For completeness, we could quote a molecular formula IF WE HAD a molecular mass determination for Ibuprofen.......but we do, it is `206.29* g*mol^-1`

Now the MOLECULAR formula is always a multiple of the empirical formula, i.e:

`206.29*g*mol^-1=nxx(13xx12.011+18xx1.00794+2xx16.0)*g*mol^-1.`

Clearly, `n=1`, and the molecluar formula is the same as the empirical formula.

Answer 2

The empirical formula for ibuprofen is `"C"_13"H"_18"O"_2"`. It is also its molecular formula.

Explanation:

The empirical formula of a compound represents the lowest whole number ratio of the elements in it. Because the percentages of carbon, hydrogen, and oxygen add up to 100%, you can directly convert them into grams.

The moles of each element must be determined. Then the mole ratio of each element is calculated by dividing by the smallest number of moles. To calculate the empirical formula, you may need to manipulate the mole ratios, such as multiplying by two.

Determine the Moles of Each Element

Divide the given mass by the molar mass of each element. The molar mass of an element is the atomic weight (relative atomic mass) on the periodic table in grams/mole, or g/mol.

`"C":` `(75.69color(red)cancel(color(black)("g")))/((12.011color(red)cancel(color(black)("g")))/(1"mol"))="6.302 mol"`

`"H":` `(8.80color(red)cancel(color(black)("g")))/((1.008color(red)cancel(color(black)("g")))/(1"mol"))="8.73 mol"`

`"O":` `(15.51color(red)cancel(color(black)("g")))/((15.999color(red)cancel(color(black)("g")))/(1"mol"))="0.9694 mol"`

Determine the Mole Ratio for Each Element.

Divide the moles of each element by the smallest number of moles.

`"C":` `(6.302color(red)cancel(color(black)("mol")))/(0.9694color(red)cancel(color(black)("mol")))=6.5`

`"H":` `(8.73color(red)cancel(color(black)("mol")))/(0.9694color(red)cancel(color(black)("mol")))=9.01`

`"O":` `(0.9694color(red)cancel(color(black)("mol")))/(0.9694color(red)cancel(color(black)("mol")))=1`

Empirical Formula

Since `6.5` is not a whole number, multiply all of the ratios by `2` so that they are all whole numbers.

`"C":` `6.5xx2=13`

`"H":` `9.01xx2=18.02~~18`

`"O":` `1xx2=2`

The empirical formula for ibuprofen is `"C"_13"H"_18"O"_2"`.

It is also its molecular formula.

The diagram below represents the structural formula for ibuprofen.