How do you solve #-\frac { 1- 2x } { x - 3} = \frac { 5- x } { 2x - 5}#?

Question

432 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-07-04T09:47:38

#x=2#

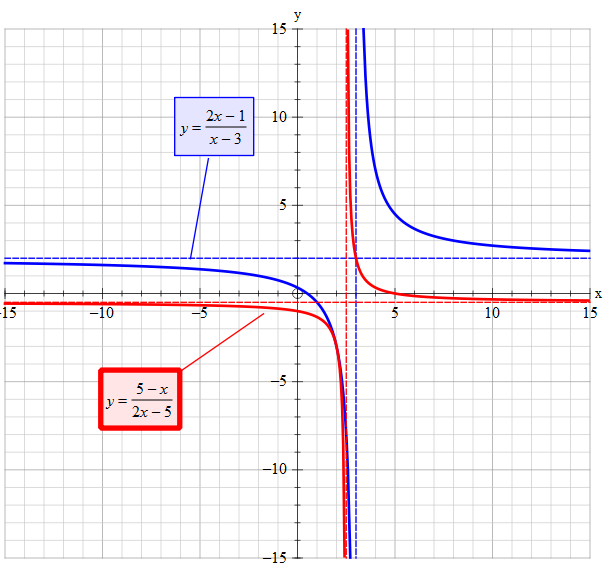
To make the LHS positive write #-(1-2x)" as "+(2x-1)# giving:

#" "(2x-1)/(x-3)" "=" "(5-x)/(2x-5)#

#" "color(green)([(2x-1)/(x-3)color(red)(xx1)]" "=" "[(5-x)/(2x-5)color(red)(xx1)])#

#color(green)([(2x-1)/(x-3)color(red)(xx(2x-5)/(2x-5))]" "=" "[(5-x)/(2x-5)color(red)(xx(x-3)/(x-3))])#

#" "((2x-1)(2x-5))/((x-3)(2x-5))" "=" "((5-x)(x-3))/((x-3)(2x-5)) #

As the denominators are the same on both sides then; if the whole equation is true then just equating the numerators to each other is also true

#" "(2x-1)(2x-5)" "=" "(5-x)(x-3) #

#" "4x^2-12x+5" "=" "-x^2+8x-15#

#" "5x^2-20x+20" "=" "0#

Divide everything by 5

#" "x^2-4x+4" "=" "0#

#" "(x-2)(x-2)" "=" "0#

#" "x" "=" "2#

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Check

#(2x-1)/(x-3)=(5-x)/(2x-5)#

LHS#->(2(2)-1)/(2-3) = 3/(-1)=-3#

RHS#->(5-2)/(2(2)-5) = 3/(-1)=-3#

Tony B