A triangle has corners at #(3 , 4 )#, #(8 ,2 )#, and #(1 ,3 )#. What is the radius of the triangle's inscribed circle?

Question

1236 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-07-19T16:21:43

The radius of the incircle is #=0.61u#

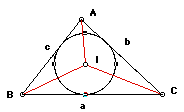
enter image source here

The length of the sides of the triangle are

#c=sqrt((8-3)^2+(2-4)^2)=sqrt(25+4)=sqrt29=5.39#

#a=sqrt((1-8)^2+(3-2)^2)=sqrt(49+1)=sqrt50=7.07#

#b=sqrt((3-1)^2+(4-3)^2)=sqrt(4+1)=sqrt5=2.24#

The area of the triangle is

#A=1/2|(x_1,y_1,1),(x_2,y_2,1),(x_3,y_3,1)|#

#=1/2(x_1(y_2-y_3)-y_1(x_2-x_3)+(x_2y_3-x_3y_2))#

#A=1/2|(3,4,1),(8,2,1),(1,3,1)|#

#=1/2(3*|(2,1),(3,1)|-4*|(8,1),(1,1)|+1*|(8,2),(1,3)|)#

#=1/2(3(2-3)-4(8-1)+1(24-2))#

#=1/2(-3-28+22)#

#=1/2|-9|=9/2#

The radius of the incircle is #=r#

#1/2*r*(a+b+c)=A#

#r=(2A)/(a+b+c)#

#=9/(14.7)=0.61#