The fundamental frequency of a closed tube is the same frequency as the 2nd harmonic of a stretched string. The mass per unit length of the string is 6.10x10^-4 kg/m. The tension in the string is 48.0N...?
What is the ratio of the length of the string to the length of the closed tube Ls/Lt?
What is the ratio of the length of the string to the length of the closed tube Ls/Lt?
1 Answer
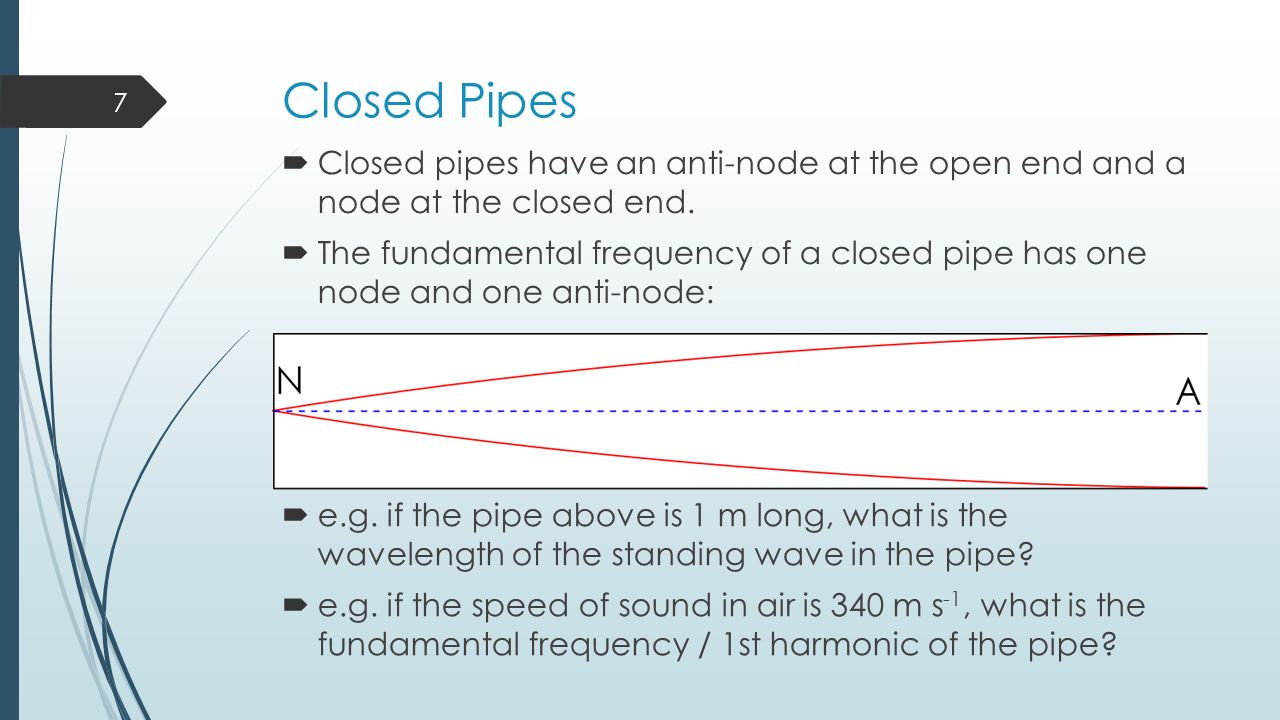
When a tune of fundamental frequency is produced in one end closed tube of length
And the frequency of the tune will be
where V is the velocity of sound

When second harmonic is produced in streched string of length
#f_s=p/(2L)sqrt(T/m)#
where
By the given condition
#f_c=f_s#
#=>V/(4l)=p/(2L)sqrt(T/m)#
#=>L/l=(2p)/Vsqrt(T/m)#
#=>L/l=(2xx2)/340sqrt(48/(6.1xx10^-4))~~3.3#

