What is the answer ?
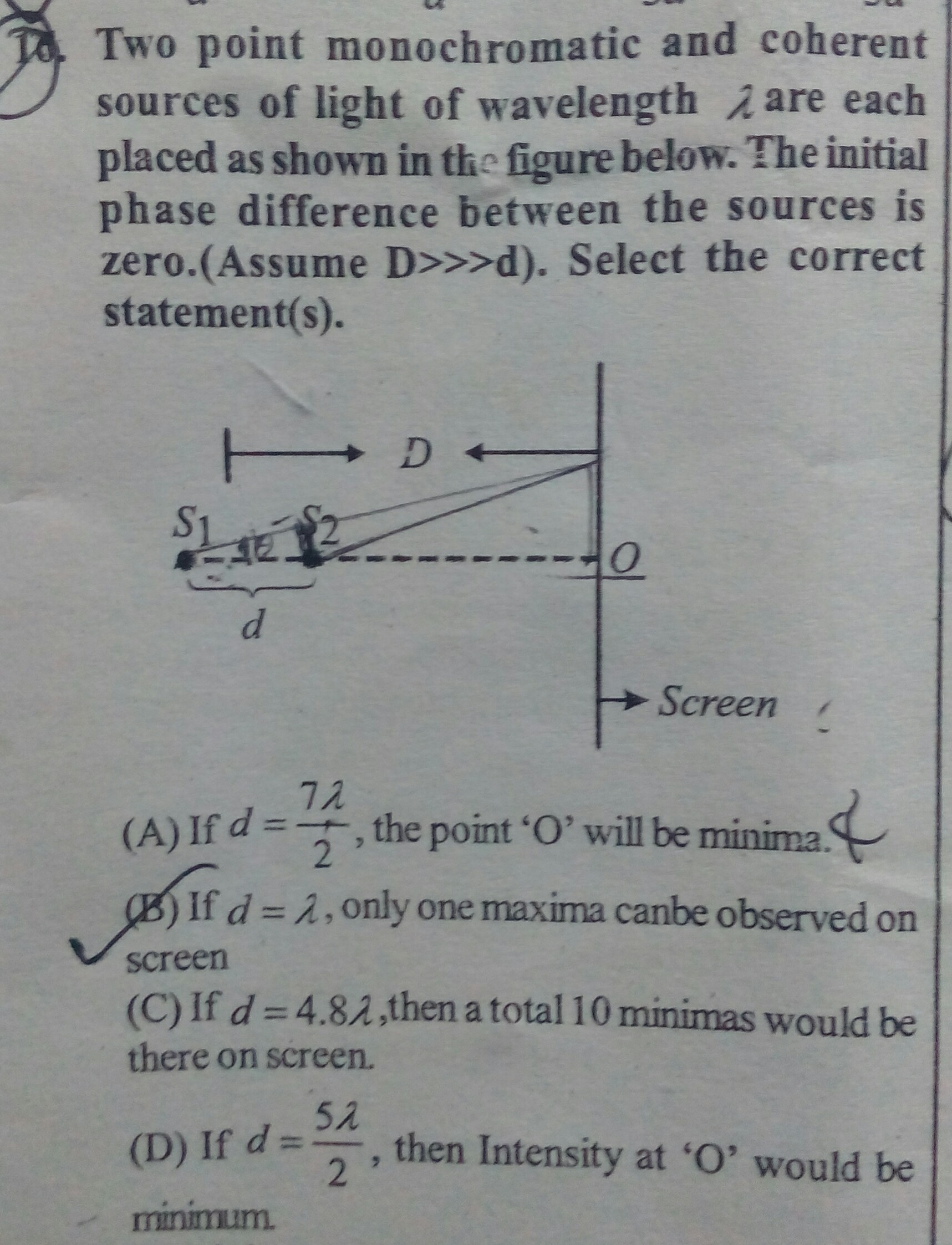
1 Answer
Condition of minima at point
d=(n+1/2)lambdad=(n+12)λ
wherenn is0,+-1,+-2,+-3....
Hence, we have minimum at
From above
Condition of maxima at point
d=nlambda
wheren is0,+-1,+-2,+-3....
Given, and from the figure
d=lambda=>n=1
It has single value. Hence there will be only one maximum at
Hence,
Consider a point
Draw a perpendicular from
As
d/2costheta
Condition for a minimum at point
d/2costheta=(n+1/2)lambda
wheren is0,+-1,+-2,+-3....
Given is
(4.8lambda)/2costheta=(n+1/2)lambda
=>costheta=1/2.4(n+1/2)
We know that
We get values of
Hence,
-.-.-.-.-.-.-.-.-.-.-.
It is interesting to investigate:
If
we get path difference as
In such a case we get
=>costheta=1/4.8(n+1/2)
We know that
We get values of
In this case
........................................
Distance of source
Similarly distance of source
Actual Path difference between the two sources at the point
