Question

Are all isosceles triangles similar?

Answer 1

See below.

Explanation:

Two triangles are only similar if all three of their angles are congruent to each other, or if two angles of one triangle are congruent with two angles of another.

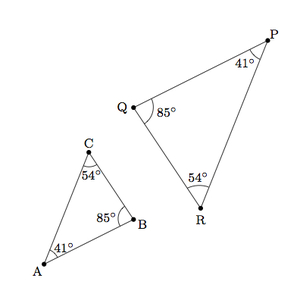
For example, these triangles are similar because their angles are congruent.

Isosceles triangles are triangles with two equal sides, and thus two equal angle measures.

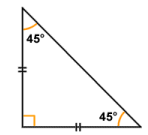
For example, both of these triangles are isosceles, since they have two equal sides and angles.

This triangle has two angles with equal measures of `75^@`.

This triangle has two angles with equal measures of `45^@`.
`color(white).`

However, since they don't have corresponding congruent angles (`75^@ != 45^@`), these triangles are not similar.

Answer 2

See a solution process below:

Explanation:

The definition of an isosceles triangle is: an isosceles triangle is a triangle that has two sides of equal length.

There is the special case where all three sides are equal which is called a equilateral triangle.

All isosceles triangles are not similar for a couple of reasons.

• The base of the triangle can stay the same but the base angles and lengths of the two equal sides can change
• The length of the two equal sides can stay the same but the measure of the angle between the two equal side will change, as will the base and the base angles.