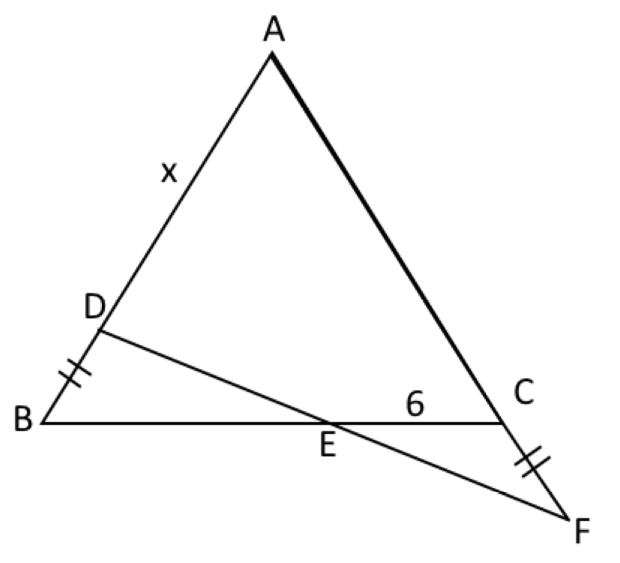
Let us solve the Problem with the help of Trigonometry.
We want to apply the Sine-Rule to #DeltaDBE, and DeltaCEF.#
Hence, we need to find, from #DeltaDBE, #
#"sides "DB, BE, /_DEB, and, /_BDE.#
Similarly, in #DeltaCEF,# we will require,
#"side "CF, "/_EFC, and, /_CEF.#
Suppose that, #BC=a, and, /_EFC=theta.#
#DeltaABC# is equilateral.
#:. AB=BC=CA=a, and, /_B=60, /_ECF=120.#
In #DeltaCEF, /_EFC+/_ECF+ /_CEF=180.#
#:. theta+120+/_CEF=180 rArr /_CEF=60-theta=/_DEB.#
Also, #BD=AB-AD=a-x, &, as CF=BD=a-x.#
Similarly, #BE=BC-EC=a-6.#
In#DeltaCEF, EC=6, CF=a-x, /_EFC=theta, /_CEF=60-theta.#
#:. (CE)/(sin/_EFC)=(CF)/(sin/_CEF).......[because," the sine-rule]."#
#rArr 6/sintheta=(a-x)/sin(60-theta)....................(1).#
Next, in #DeltaDBC, DB=a-x, BE=a-6, /_DEB=60-theta, &#
#/_BDE=180-(/_DBE+/_DEB)=180-(60+60-theta)=60+theta.#
#:.," by the sine-rule, "(DB)/(sin/_DEB)=(BE)/(sin/_BDE).#
#rArr (a-x)/sin(60-theta)=(a-6)/sin(60+theta)..............(2).#
#(1), &, (2) rArr 6/sintheta=(a-6)/sin(60+theta), or,#
#sin(60+theta)/sintheta=(a-6)/6.#
#:. (sin60costheta+cos60sintheta)/sintheta=a/6-6/6.#
#:.sqrt3/2*costheta/sintheta+1/2*sintheta/sintheta=a/6-1.#
#:. sqrt3/2*cottheta+1/2+1=a/6, or, a=3sqrt3cottheta+9....(3).#
Now, from #(1), 6/sintheta=(a-x)/sin(60-theta).#
#:. sin(60-theta)/sintheta=(a-x)/6.#
#:. (sin60costheta-cos60sintheta)/sintheta=(a-x)/6.#
#:. sqrt3/2*costheta/sintheta-1/2*sintheta/sintheta=(a-x)/6.#
#:. 6(sqrt3/2*cottheta-1/2)=(a-x).#
#:. 3sqrt3cottheta-3-a=-x.#
Finally, by #(3),# then,
#x=a+3-3sqrt3cottheta=(3sqrt3cottheta+9)+3-3sqrt3cottheta.#
# rArr x=12,# as Respected CW has already obtained!
Enjoy Maths.!