Question #cbeb6
1 Answer
# x(alpha) = (10.4)^2/g \ sin2alpha#
Maximum horizontal distance is achieved when
Explanation:
For Physics or Mechanics you should learn the "suvat" equations for motion under constant acceleration:
#{: (v=u+at, " where ", s="displacement "(m)), (s=ut+1/2at^2, , u="initial speed "(ms^-1)), (s=1/2(u+v)t, , v="final speed "(ms^-1)), (v^2=u^2+2as, , a="acceleration "(ms^-2)), (s=vt-1/2at^2, , t="time "(s)) :} #
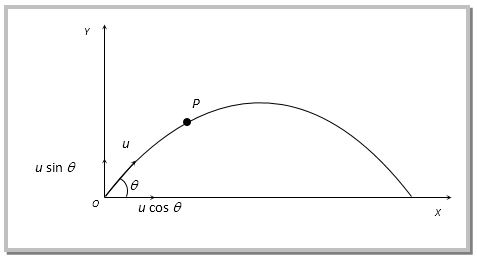
Horizontal Motion
The projectile will move under constant speed (NB we can still use "suvat" equation with a=0).
The projectile will travel a distance
# { (s=,x,m),(u=,10.4 cos alpha,ms^-1),(v=,"Not Required",ms^-1),(a=,0,ms^-2),(t=,T,s) :} #
So applying
# x = 10.4cosalphaT# ..... [A]
Vertical Motion
The projectile travels under constant acceleration due to gravity. Its total displacement will be zero at the point where the projectile reaches ground level, and travels its maximum distance, and we consider the same time interval,
# { (s=,0,m),(u=,10.4sin alpha,ms^-1),(v=,"Not Required",ms^-1),(a=,-g,ms^-2),(t=,T,s) :} #
Applying
# 0 = 10.4sinalphaT+1/2(-g)T^2 #
# :. gT^2 - 20.8sinalphaT = 0#
# :. T(gT - 20.8sinalpha) = 0#
Leading to two possible solutions for
# T = 0 # (Where the projectile leaves the ground)
# T = (20.8sinalpha)/g # (Where the projectile returns to the ground
Horizontal Distance Travelled
Using this value of
# x(alpha) = 10.4(cosalpha) * (20.8sinalpha)/g#
# \ \ \ \ \ \ \ = 10.4(cosalpha) * (2*10.4)(sinalpha)/g#
# \ \ \ \ \ \ \ = ((10.4)^2(2sinalphacosalpha))/g#
# \ \ \ \ \ \ \ = (10.4)^2/g \ sin2alpha# ..... [B]
So we have an expression giving the horizontal distance travelled as a function of the launch angle,
# x(alpha) = (10.4)^2/g \ sin2alpha# ..... [B]
Consistent with the given solution.
We get a maximum distance at a critical point of [B], so let us find any critical points. It is vital to remember that when dealing with calculus of trigonometric functions that we must work in radians. And for this problem we require,
Differentiating [B] wrt
# (dx)/(d alpha) = (10.4)^2/g \ (2cos2alpha)#
At a critical point, this derivative is zero, giving:
# (dx)/(d alpha) = 0 => cos2alpha = 0#
# :. 2alpha = pi/2 \ \ \ \ # for#0 le 2alpha le pi #
# :. alpha = pi/4 \ \ \ \ \ \ # for#0 le alpha le pi/2 #
Which confirms that the maximum horizontal distance is achieved when

