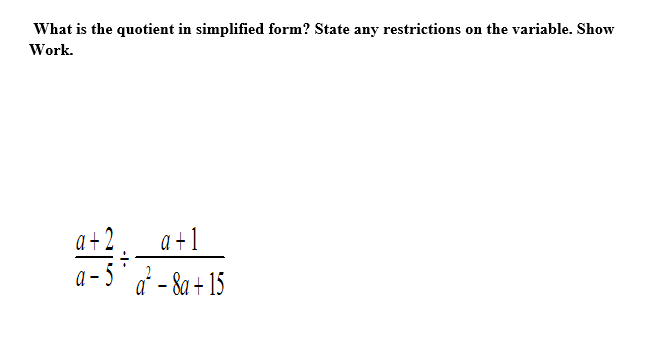
We have: #frac(a + 2)(a - 5) div frac(a + 1)(a^(2) - 8 a + 15)#
#= frac(a + 2)(a - 5) times frac(a^(2) - 8 a + 15)(a + 1)#
#= frac(a + 2)(a - 5) times frac(a^(2) - 3 a - 5 a + 15)(a + 1)#
#= frac(a + 2)(a - 5) times frac(a (a - 3) - 5 (a - 3))(a + 1)#
#= frac(a + 2)(a - 5) times frac((a - 3)(a - 5))(a + 1)#
#= frac((a + 2)(a - 3)(a - 5))((a + 1)(a - 5))#
#= frac((a + 2)(a - 3))(a + 1)#
Now, let's find the restricted values of #a#.
The denominators of the original expression contained #a - 5# and #a^(2) - 8 a + 15#, which factorised into #(a - 3)(a - 5)#.
These values cannot be equal to zero, i.e. #a ne 5# and #a ne 3, 5#.
Combining these results we get #a ne 3, 5#.
Also, in the last line, the denominator is #a + 1 ne 0#, or #a ne - 1#.
Therefore, the restricted values of #a# are #- 1, 3,# and #5#.