Question #a51c8
1 Answer
The point
The point
Explanation:
Firstly, here are the steps for the differentiation.
Differentiate everything (implicitly) with respect to
#2x - 2 (y + x frac{dy}{dx}) + 2y frac{dy}{dx} + 6 - 10 frac{dy}{dx} = 0#
Bring all the terms containing
#2x - 2y + 6 = 2x frac{dy}{dx} - 2y frac{dy}{dx} + 10 frac{dy}{dx}#
Remove the common multiple of 2.
#x - y + 3 = x frac{dy}{dx} - y frac{dy}{dx} + 5 frac{dy}{dx}#
Make
#frac{dy}{dx} = frac{ x - y + 3 }{x - y + 5}#
Which is what you have gotten.
To find the point(s) with horizontal tangent line, you should look for points
#frac{dy}{dx} = frac{ x - y + 3 }{x - y + 5} = 0#
which simplifies to
#y = x + 3#
Bear in mind that the point
#x^2 - 2xy + y^2 + 6x - 10y + 25 = 0#
Solve this simultaneous equation by substitution.
#x^2 - 2x(x + 3) + (x + 3)^2 + 6x - 10(x + 3) + 25 = 0#
Expanding the terms reveal that the
#4 - 4x = 0#
#x = 1#
#y = 1 + 3 = 4#
The point
To find the point(s) with vertical tangent line, you should look for points
This is due to denominator being zero for a certain point
#x - y + 5 = 0#
or
#y = x + 5#
Again, the point
#x^2 - 2xy + y^2 + 6x - 10y + 25 = 0#
Substituting directly, we get the equation below.
#x^2 - 2x(x + 5) + (x + 5)^2 + 6x - 10(x + 5) + 25 = 0#
After expansion, we have a first order polynomial again.
#-4x = 0#
#x = 0#
#y = 5#
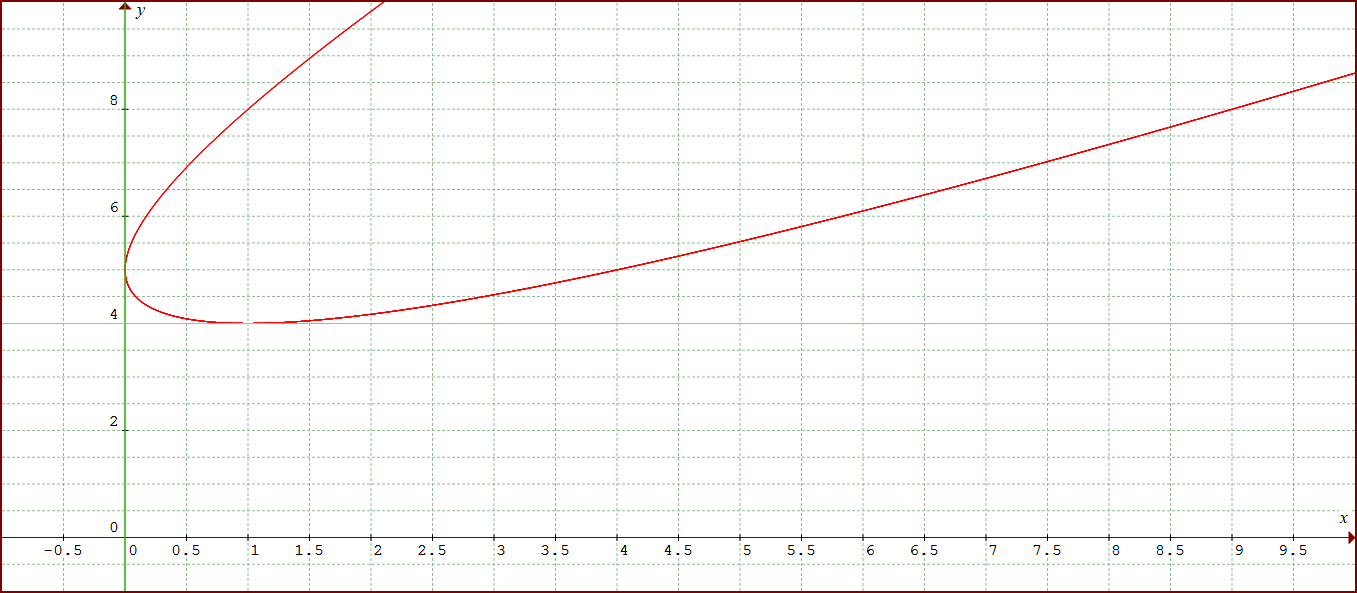
The point
Refer to the graph below. The horizontal tangent is in cyan while the vertical tangent is in lime.