Question #e56e4
1 Answer
Explanation:
For starters, the frequency you have here is way too low to match that of a gamma ray. Gamma rays have frequencies that are
In fact, the frequency given to you matches that of a photon in the infrared portion of the EM spectrum.
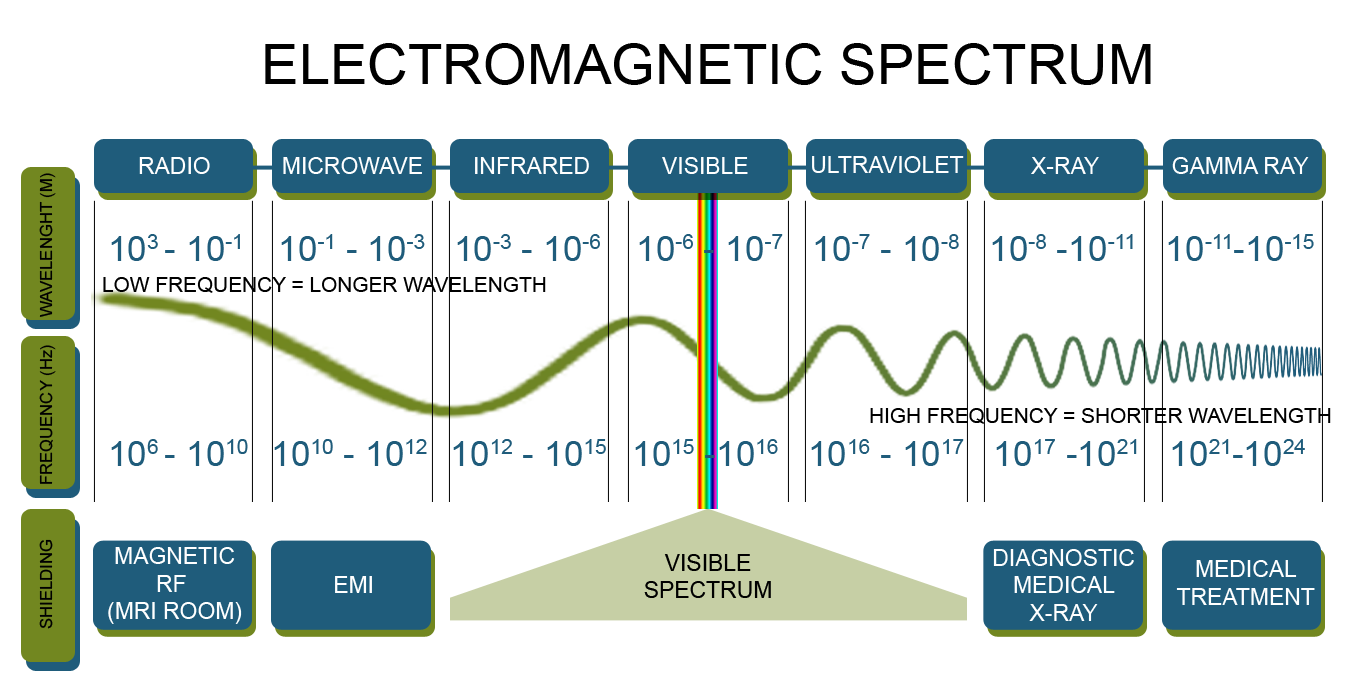
I'll show you hot to find the energy of this photon, but keep in mind that you are not dealing with a gamma ray here!
The most important thing to remember about the energy of a photon is that it is directly proportional to its frequency as described by the Planck - Einstein relation.
#E =h * nu#
Here
#E# is the energy of the photon#h# is Planck's constant, equal to#6.626 * 10^(-34)"J s"# #nu# is the frequency of the photon
This basically means that a photon that has a high frequency will also have a high energy. In other words, the higher the frequency of the photon, the higher its energy.
Now, notice that the frequency of the photon is given to you in hertz,
#"1 Hz" = "1 s"^(-1)#
Plug in the value you have for the frequency of the photon and solve for
#E = 6.626 * 10^(-34)color(white)(.)"J" color(red)(cancel(color(black)("s"))) * 1.0 * 10^(13)color(red)(cancel(color(black)("s"^(-1))))#
#E = color(darkgreen)(ul(color(black)(6.6 * 10^(-21)color(white)(.)"J")))#
The answer is rounded to two sig figs, the number of sig figs you have for the frequency of the photon.

