Question

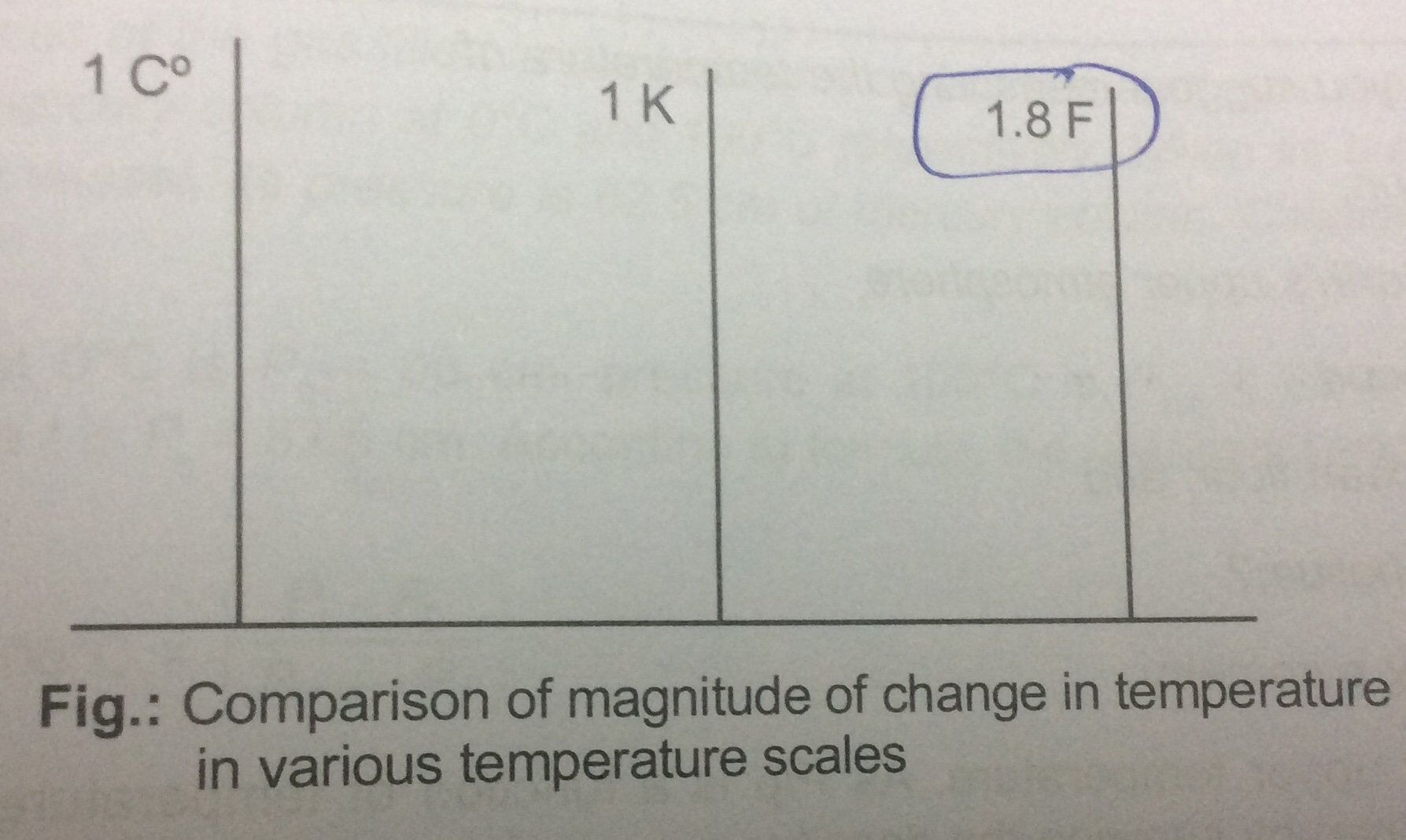
Can someone explain me how this 1.8F came? I know that change in temperature in Celsius scale is equal to change in temperature in Kelvin scale. But, what about Fahrenheit scale?

Answer 1

See the explanation below

Explanation:

To convert from the Centigrade Scale to the Fahrenheit Scale, we apply the equation

`(F-32)/(C)=9/5`

as the Fahrenheit Scale ranges from `32^@F` to `212^@F` and

The Centigrade Scale ranges from `0^@C` to `100 ^@C`

Therefore,

`(212-32)^@F=(100-0)^@C`

`180^@F=100^@C`

`1^@C=180/100=1.8^@F`

Answer 2

In constructing a device and a scale to measure temperature, phase transition of water was used as reference.

Centigrade: In the centigrade scale, the temperature interval between the melting point of ice and boiling point of water was uniformly divided into 100 intervals. So the melting point of ice and boiling point of ice are 100 units away in this scale.

Fahrenheight: In the Fahrenheit scale, the dial was constructed to sweep exactly `180^o` between melting point of ice and boiling point of water. The temperature interval between these were uniformly divided into 180 units. So the melting point of ice and boiling point of ice are 180 units away in this scale.

Therefore, `\quad \Delta T_F = 180^0 F` corresponds to `\DeltaT_C = 100^oC`

Since the temperature intervals were divided uniformly in both scales, these two scales must be linearly related to each other.

`T_F = aT_C + b`

`a = (\DeltaT_F)/(\Delta T_C) = (180^oF)/(100^oC) = 9/5`
`b = T_F(T_C=0^0C) = 32^0F`

Therefore,
`T_F = 9/5T_C + 32`

Now, coming to the temperature differences,

`\Delta T_F = 9/5\DeltaT_C = 1.8 \DeltaT_C = 1.8\DeltaT_K`