This question has so many parts that I am not giving detailed explanations.
a- The chemical equation
"NH"_3 + "HCl" → "NH"_4"Cl"
b- Volume of "HCl" at the equivalence point
"Moles of NH"_3 = 0.050 color(red)(cancel(color(black)("L NH"_3))) × "0.1 mol NH"_3/(1 color(red)(cancel(color(black)("L NH"_3)))) = "0.005 mol NH"_3
"Moles of HCl" = 0.005 color(red)(cancel(color(black)("mol NH"_3))) × "1 mol HCl"/(1 color(red)(cancel(color(black)("mol NH"_3)))) = "0.005 mol HCl"
"Volume of HCl" = 0.005 color(red)(cancel(color(black)("mol HCl"))) × "1 L HCl"/(0.1 color(red)(cancel(color(black)("mol HCl")))) = "0.05 L HCl" = "50 mL HCl"
c- pH at different volumes
(i) pH at 0 mL
color(white)(mmmmmml)"NH"_3 + "H"_2"O" ⇌ "NH"_4^"+" + "OH"^"-"
"I/mol·L"^"-1": color(white)(mll)0.1color(white)(mmmmmmll)0color(white)(mmm)0
"C/mol·L"^"-1": color(white)(mll)"-"xcolor(white)(mmmmmm)"+"xcolor(white)(mml)"+"x
"E/mol·L"^"-1": color(white)(m)"0.1-"xcolor(white)(mmmmmm)xcolor(white)(mmm)x
K_text(b) = (["NH"_4^"+"]["OH"^"-"])/(["NH"_3]) = x^2/(0.1-x) = 1.76 × 10^"-5"
x^2 = 0.1 × 1.76 × 10^"-5" = 1.8 × 10^"-6"
x = 1.3 × 10^"-3"
["OH"^"-"] = xcolor(white)(l) "mol/L" = 1.3 × 10^"-3" color(white)(l)"mol/L"
"pOH" = "-log"(1.3 × 10^"-3") = 2.88
"pH = 14.00 - 2.88 = 11.12"
(ii) pH at 10 mL "HCl"
color(white)(mmmmml)"NH"_3 + "H"_3"O"^"+" → "NH"_4^"+"
"I/mol": color(white)(mll)0.005color(white)(ml)0.001color(white)(mmm)0
"C/mol": color(white)(m)"-0.001"color(white)(m)"-0.001"color(white)(m)"+0.001"
"E/mol": color(white)(ml)0.004color(white)(mml)0color(white)(mmm)0.001
This is a buffer!
"p"K_text(b) = "-log"(1.76 × 10^"-5") = 4.75
"pOH" = "p"K_text(b) + log((["NH"_4^"+"])/(["NH"_3])) = 4.75 + log(0.001/0.004) = 4.75 - 0.60 = 4.15
"pH" = 14.00 - 4.15 = 9.85"
(iii) pH at the equivalence point.
We have neutralized all the "NH"_3, so we have 0.005 mol "NH"_4^"+" in 100 mL solution.
["NH"_4^"+"] = "0.05 mol/L"
color(white)(mmmmmmll)"NH"_4^"+" + "H"_2"O" ⇌ "NH"_3 +"H"_3"O"^"+"
"I/mol·L"^"-1": color(white)(mll)0.05color(white)(mmmmmmll)0color(white)(mmm)0
"C/mol·L"^"-1": color(white)(mll)"-"xcolor(white)(mmmmmmll)"+"xcolor(white)(mm)"+"x
"E/mol·L"^"-1": color(white)(m)"0.05-"xcolor(white)(mmmmmm)xcolor(white)(mmm)x
K_text(a) = K_text(w)/K_text(b) = (1.00 × 10^"-14")/(1.76 × 10^"-5") = 5.68 × 10^"-10"
K_text(a) = (["NH"_3]["H"_3"O"^"+"])/(["NH"_4^"+"]) = x^2/(0.05-x) = 5.68 × 10^"-10"
x^2 = 0.05 × 5.68 × 10^"-10" = 2.8 × 10^"-11"
x = 5.3 × 10^"-6"
["H"_3"O"^"+"] = xcolor(white)(l) "mol/L" = 5.3 × 10^"-6" color(white)(l)"mol/L"
"pH" = "-log"(5.3 × 10^"-6") = 5.27
(iv) pH at 60 mL "HCl"
We have added 0.006 mol of "HCl", and 0.005 mol have been neutralized by the "NH"_3.
Thus, we have 0.001 mol of "HCl" in 110 mL of solution.
["H"_3"O"^"+"] = "0.001 mol"/"0.110 L" = 9.1 × 10^"-3" color(white)(l)"mol/L"
"pH = "=log"(9.1 × 10^"-3") = 2.04
We leave it as an exercise for the student to calculate the pH at other volumes of "HCl".
d- Titration table
Here's a table I created in Excel (not all the volumes are yours).
ulbb("V/mL"color(white)(m)"pH")
color(white)(m)0.0color(white)(ml)11.12
color(white)(ll)12.5color(white)(mll) 9.72
color(white)(ll)25.0color(white)(mll) 9.24
color(white)(ll)45.0color(white)(mll) 8.29
color(white)(ll)50.0color(white)(mll) 5.27
color(white)(ll)55.0color(white)(mll) 2.32
color(white)(ll)75.0color(white)(mll) 1.70
color(white)(l)100.0color(white)(ml)1.48
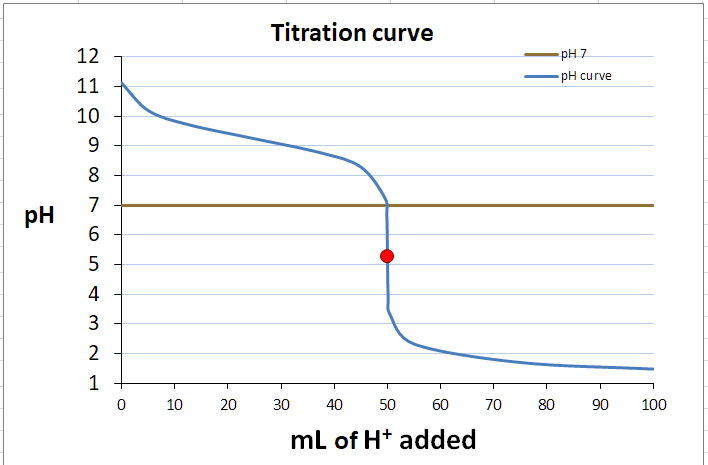
e- Construct the titration curve
Here's what I got.
 pH Plot
pH Plot
f- Identify the end point on the curve
It is approximately at the position of the red dot.

 pH Plot
pH Plot 
