In a circle of diameter 40 cm, the length of a chord is 20 cm. Find the length of minor arc of the chord?
1 Answer
Dec 13, 2017
I got
Explanation:
Let us have a look at the diagram:
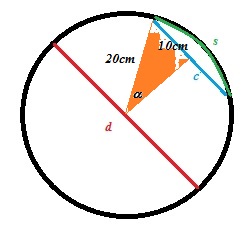
and consider the orange right triangle where the hypotenuse is equal to the radius
We can evaluate the angle
so that the entire angle
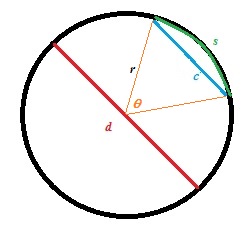
in radians this angle is:
We can use this value in radians into:
to find the length of the arc

