A spherical shell of radius #R# and uniformly charged with charge #Q# is rotating about its axis with frequency #f#. Find the magnetic moment of the sphere?
1 Answer
Uniformly charged spherical shell of radius
Hence it has surface charge density
#sigma=Q/(4πR^2)#
It rotates about its axis with frequency
Suppose the angular velocity
To find the magnetic moment of spinning shell we can divide
it into infinitesimal charges.
Using spherical polar coordinates
Here
#dq=sigmadS=sigmaR^2sinthetad thetadphi#
Current in the ring is given by
#dI=(dq)xxf=(sigmaR^2sinthetad thetadphi)xxomega/(2pi)#
#=>dI=(omegasigmaR^2sinthetad thetadphi)/(2pi)#
Now the magnetic dipole moment of ring is given by the expression in terms of position vector
#dvecm=1/2int_"ring"vecrxxvecJ#
#=>dvecm=1/2dIint_"ring"vecrxxdvecl#
where#dvecl# is element length of the ring.
Line integral becomes equal to the circumference of ring
#:.dvecm=dI(piR^2sin^2theta)hatz#
Inserting value of
#dvecm=(omegasigmaR^2sinthetad thetadphi)/(2pi)(piR^2sin^2theta)hatz#
#=>dvecm=(vecomegasigmaR^4sin^3thetad thetadphi)/2#
Total magnetic moment is integral with respect to both variables within respective limits
#vecm=(vecomegasigmaR^4)/2int_0^pisin^3thetad thetaint_0^(2pi)dphi#
#=>vecm=(vecomegasigmaR^4)/2xx4/3xx2pi#
#=>vecm=4/3pisigmaR^4vecomega#
Rewriting in terms of charge
#vecm=4/3pi(Q/(4πR^2))R^4vecomega#
#vecm=Q/3R^2vecomega#
.-.-.-.-.-.-.-.-
Reference figure for
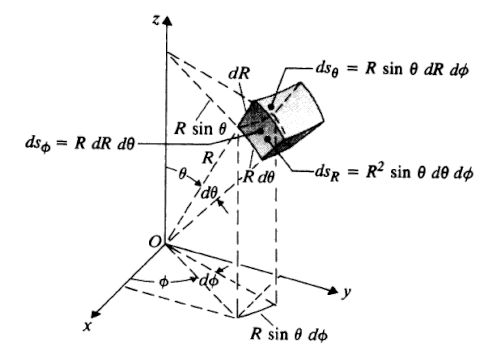
Using online integral calculator
#int_0^pisin^3thetad theta=|cos^3theta/3-costheta|_0^pi=4/3#
