A balanced lever has two weights on it, the first with mass #12 kg # and the second with mass #4 kg#. If the first weight is # 7 m# from the fulcrum, how far is the second weight from the fulcrum?
2 Answers
Jan 14, 2018
The distance is
Explanation:
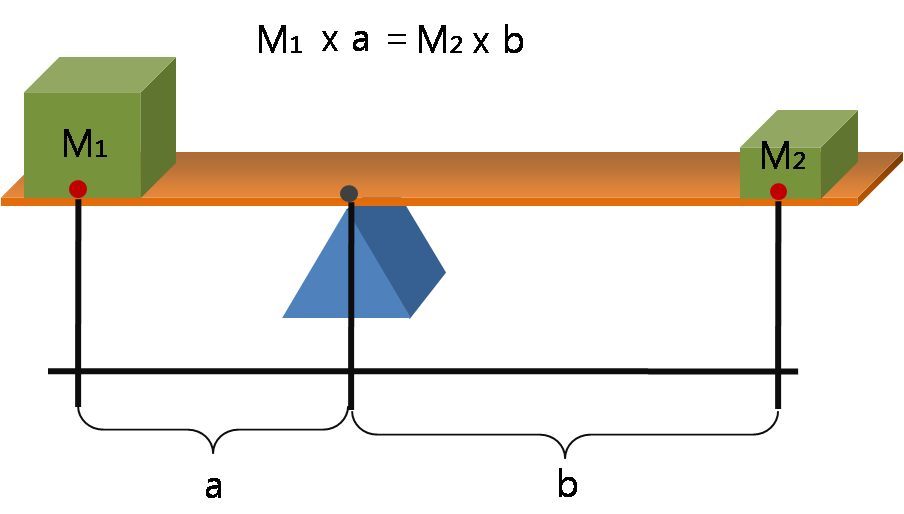
The mass
The mass
The distance
Taking moments about the fulcrum
Jan 14, 2018
Explanation:
the lever is balanced, meaning that the moments on either side are equal.
the moment is the force of the weight multiplied by the distance of the weight from the fulcrum.
moment (Nm)
the moment of the weight with mass
note:
weight (in newtons) = mass * gravitational field strength (~9.81)
mass
mass


